Coxeter group

A Coxeter group is a mathematical group generated by reflections in Euclidean space. These groups are ubiquitous in mathematics and geometry. The finite Coxeter groups are precisely the finite Euclidean reflection groups. Consequently, the symmetry groups of all the regular polytopes are finite Coxeter groups. The Weyl groups of root systems are also all special cases of finite Coxeter groups.

Coxeter groups are named for the geometer H. S. M. Coxeter.

Contents

1 Definition 2 An example 3 Finite Coxeter groups 4 Symmetry groups of regular polytopes 5 Affine Weyl groups 6 Hyperbolic Coxeter groups 7 See also

Definition

Formally, a Coxeter group can be defined as a group with the presentation

<math>\left\langle r_1,r_2,\ldots,r_n \mid (r_ir_j)^{m_{ij}}=1\right\rangle<math>

where m_ii = 1 and m_ij ≥ 2 for i ≠ j. The condition m_ij = ∞ means no relation of the form (r_i r_j)^m should be imposed. The relation m_ii = 1 means that (r_i)² = 1 for all i (i.e. the generators are involutions). Under the condition that (r_i)² = 1 one can show that

<math>(r_ir_j)^{m_{ij}} = 1 \;\Leftrightarrow\; (r_jr_i)^{m_{ij}} = 1<math>

It is then convenient to regard m_ij as the entries of a n×n symmetric matrix with 1's on the diagonal called the Coxeter matrix.

The Coxeter matrix can be encoded as a Coxeter graph in which the vertices stand for generator subscripts, i and j are connected if and only if m_ij ≥ 3, and the edge is labelled with the value of m_ij whenever it is 4 or greater. In particular, two generators commute if and only if they are not connected by an edge. This is because when

x² = y² = 1,

we have

yx = xy if and only if xyxy = xxyy,

i.e., if and only if

(xy)² = 1.

In particular, if a Coxeter graph has two or more connected components, the associated group is the direct product of the groups associated to the individual components.

An example

The graph in which vertices 1 through n are placed in a row with each vertex connected by an unlabelled edge to its immediate neighbors gives rise to the symmetric group S_n+1; the generators correspond to the transpositions (1 2), (2 3), ... (n n+1). Two non-consecutive transpositions always commute, while (k k+1) (k+1 k+2) gives the 3-cycle (k k+1 k+2). Of course this only shows that S_n+1 is a quotient group of the Coxeter group, but it is not too difficult to check that equality holds.

Finite Coxeter groups

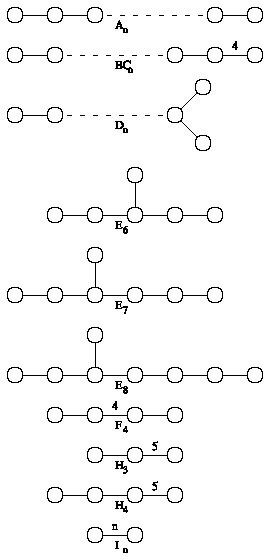
Every Weyl group can be realized as a Coxeter group. The Coxeter graph can be obtained from the Dynkin diagram by replacing every double edge with an edge labelled 4 and every triple edge by an edge labelled 6. The example given above corresponds to the Weyl group of the root system of type A_n. The Weyl groups include most of the finite Coxeter groups, but there are additional examples as well. The following list gives all connected Coxeter graphs giving rise to finite groups:

Comparing this with the list of simple root systems, we see that B_n and C_n give rise to the same Coxeter group. Also, G₂ appears to be missing, but it is present under the name I₂(6). The additions to the list are H₃, H₄, and the I₂(p).

Some properties of the finite Coxeter groups are given in the following table:

Type	Rank	Order	Polytope
An	n	(n + 1)!	n-simplex
Bn = Cn	n	2n n!	n-cube / n-cross-polytope
Dn	n	2n−1 n!
I2(p)	2	2p	p-gon
H3	3	120	icosahedron / dodecahedron
F4	4	1152	24-cell
H4	4	14400	120-cell / 600-cell
E6	6	51840	E6 polytope
E7	7	2903040
E8	8	696729600

Symmetry groups of regular polytopes

All symmetry groups of regular polytopes are finite Coxeter groups. The dihedral groups, which are the symmetry groups of regular polygons, form the series I₂(p). The symmetry group of a regular n-simplex is the symmetric group S_n+1, also known as the Coxeter group of type A_n. The symmetry group of the n-cube is the same as that of the n-cross-polytope, namely BC_n. The symmetry group of the regular dodecahedron and the regular icosahedron is H₃. In dimension 4, there are three special regular polytopes, the 24-cell, the 120-cell, and the 600-cell. The first has symmetry group F₄, while the other two have symmetry group H₄.

The Coxeter groups of type D_n, E₆, E₇, and E₈ are the symmetry groups of certain semiregular polytopes.

Affine Weyl groups

The affine Weyl groups form a second important series of Coxeter groups. These are not finite themselves, but each contains a normal abelian subgroup such that the corresponding quotient group is finite. In each case, the quotient group is itself a Weyl group, and the Coxeter graph is obtained from the Coxeter graph of the Weyl group by adding an additional vertex and one or two additional edges. For example, for n ≥ 2, the graph consisting of n+1 vertices in a circle is obtained from A_n in this way, and the corresponding Coxeter group is the affine Weyl group of A_n. For n = 2, this can be pictured as the symmetry group of the standard tiling of the plane by equilateral triangles.

Hyperbolic Coxeter groups

There are also hyperbolic Coxeter groups describing reflection groups in hyperbolic geometry.

See also

• Artin group
• Weyl groupja:コクセター群