Complex number
|
|
In mathematics, the complex numbers are an extension of the real numbers by the inclusion of the imaginary unit i, satisfying i^2 = -1. Every complex number can be written in the form x+iy, where <math>x<math> and <math>y<math> are real numbers called the real part and the imaginary part of the complex number, respectively. Pairs of complex numbers can be added, subtracted, multiplied, and divided in a manner similar to that of real numbers. Formally, one says that the set of all complex numbers forms a field.
The set of all complex numbers is usually denoted by C, or in blackboard bold by <math>\mathbb{C}<math>.
The complex numbers, in contrast to the real numbers, have the primary advantage that they are algebraically closed, that is, all non-constant polynomials with complex coefficients have roots in the complex numbers. This result is known as the fundamental theorem of algebra.
In mathematics, the term "complex" when used as an adjective means that the field of complex numbers is the underlying number field considered, for example complex matrix, complex polynomial and complex Lie algebra.
Definition
Formally, the complex numbers are defined as ordered pairs of real numbers (a, b) together with the operations:
- <math> ( a , b ) + ( c , d ) = ( a + c , b + d ) \,<math>
- <math> ( a , b ) \cdot ( c , d ) = ( ac - bd , bc + ad ). \,<math>
So defined, the complex numbers form a field, the complex number field, denoted by C (or <math>\mathbb{C}<math> in blackboard bold).
We identify the real number a with the complex number (a, 0), and in this way the field of real numbers R becomes a subfield of C. The imaginary unit i is the complex number (0, 1).
In C, we have:
- additive identity ("zero"): (0, 0)
- multiplicative identity ("one"): (1, 0)
- additive inverse of (a,b): (−a, −b)
- multiplicative inverse of non-zero (a, b): <math>\left({a\over a^2+b^2},{-b\over a^2+b^2}\right).<math>
C could also be defined as the topological closure of algebraic numbers and the algebraic closure of R.
Geometry
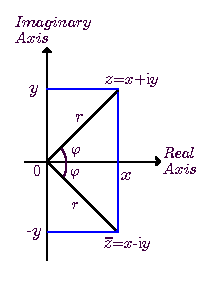
A complex number can also be viewed as a point or a position vector on the two dimensional Cartesian coordinate system. This representation is called the complex plane or Argand diagram. In the figure, we have
- <math> z = x + \mathrm{i}y = r (\cos \phi + \mathrm{i}\sin \phi ). \,<math>
The latter expression is sometimes shorthanded as r cis φ, where r = |z| is called the absolute value of z and φ = arg(z) is called the complex argument of z. However, Euler's formula states that eiφ = cisφ. The exponential form gives us a better insight than the shorthand rcisφ, which is almost never used in serious mathematical articles. By simple trigonometric identities, we see that
- <math>r_1 e^{\mathrm{i}\phi_1} \cdot r_2 e^{\mathrm{i}\phi_2}
= r_1 r_2 e^{\mathrm{i}(\phi_1 + \phi_2)} \,<math> and that
- <math>\frac{r_1 e^{\mathrm{i}\phi_1}}
{r_2 e^{\mathrm{i}\phi_2}} = \frac{r_1}{r_2} e^{\mathrm{i} (\phi_1 - \phi_2)}. \,<math>
Now the addition of two complex numbers is just the vector addition of two vectors, and the multiplication with a fixed complex number can be seen as a simultaneous rotation and stretching.
Multiplication with i corresponds to a counter clockwise rotation by 90 degrees. The geometric content of the equation i2 = −1 is that a sequence of two 90 degree rotation results in a 180 degree rotation. Even the fact (−1) · (−1) = +1 from arithmetic can be understood geometrically as the combination of two 180 degree turns.
Absolute value, conjugation and distance
Recall that the absolute value (or modulus or magnitude) of a complex number z = r eiφ is defined as |z| = r. Algebraically, if z = a + ib, then |z| = √(a² + b² ).
One can check readily that the absolute value has three important properties:
- <math> | z + w | \leq | z | + | w | \,<math>
- <math> | z w | = | z | \; | w | \,<math>
- <math> | z / w | = | z | / | w | \,<math>
for all complex numbers z and w. By defining the distance function d(z, w) = |z − w| we turn the complex numbers into a metric space and we can therefore talk about limits and continuity. The addition, subtraction, multiplication and division of complex numbers are then continuous operations. Unless anything else is said, this is always the metric being used on the complex numbers.
The complex conjugate of the complex number z = a + ib is defined to be a - ib, written as <math>\bar{z}<math> or z*. As seen in the figure, <math>\bar{z}<math> is the "reflection" of z about the real axis. The following can be checked:
- <math>\overline{z+w} = \bar{z} + \bar{w}<math>
- <math>\overline{zw} = \bar{z}\bar{w}<math>
- <math>\overline{(z/w)} = \bar{z}/\bar{w}<math>
- <math>\bar{\bar{z}}=z<math>
- <math>\bar{z}=z<math> iff z is real
- <math>|z|=|\bar{z}|<math>
- <math>|z|^2 = z\bar{z}<math>
- <math>z^{-1} = \bar{z}|z|^{-2}<math> if z is non-zero.
The latter formula is the method of choice to compute the inverse of a complex number if it is given in rectangular coordinates.
That conjugate commutes with all the algebraic operations (and many functions; e.g. <math>\sin\bar z=\overline{\sin z}<math>) is rooted in the ambiguity in choice of i (−1 has two square roots); note, however, that conjugate is not differentiable (see holomorphic).
The complex argument of z=reiφ is φ. Note that the complex argument is unique modulo 2π, that is, any two values of the complex argument differ by an integer multiple of 2π.
Complex number division
Given a complex number (a + ib) which is to be divided by another complex number (c + id) whose magnitude is non-zero, there are two ways to do this. The first way has already been implied: to convert both complex numbers into exponential form, from which their quotient is easy to derive. The second way is to express the division as a fraction, then to multiply both numerator and denominator by the complex conjugate of the denominator. This causes the denominator to simplify into a real number:
- <math> {a + \mathrm{i}b \over c + \mathrm{i}d} = {(a + \mathrm{i}b) (c - \mathrm{i}d) \over (c + \mathrm{i}d) (c - \mathrm{i}d)} = {(ac + bd) + \mathrm{i}(bc - ad) \over c^2 + d^2} <math>
- <math> = \left({ac + bd \over c^2 + d^2}\right) + \mathrm{i}\left( {bc - ad \over c^2 + d^2} \right). <math>
Matrix representation of complex numbers
While usually not useful, alternative representations of complex fields can give some insight into their nature. One particularly elegant representation interprets every complex number as 2×2 matrix with real entries which stretches and rotates the points of the plane. Every such matrix has the form
- <math>
\begin{pmatrix}
a & -b \\ b & \;\; a
\end{pmatrix} <math>
with real numbers a and b. The sum and product of two such matrices is again of this form. Every non-zero such matrix is invertible, and its inverse is again of this form. Therefore, the matrices of this form are a field. In fact, this is exactly the field of complex numbers. Every such matrix can be written as
- <math>
\begin{pmatrix}
a & -b \\ b & \;\; a
\end{pmatrix} = a \begin{pmatrix}
1 & \;\; 0 \\ 0 & \;\; 1
\end{pmatrix} + b \begin{pmatrix}
0 & -1 \\ 1 & \;\; 0
\end{pmatrix} <math> which suggests that we should identify the real number 1 with the matrix
- <math>
\begin{pmatrix}
1 & \;\; 0 \\ 0 & \;\; 1
\end{pmatrix} <math> and the imaginary unit i with
- <math>
\begin{pmatrix}
0 & -1 \\ 1 & \;\; 0
\end{pmatrix} <math>
a counter-clockwise rotation by 90 degrees. Note that the square of this latter matrix is indeed equal to −1.
The absolute value of a complex number expressed as a matrix is equal to the square root of the determinant of that matrix. If the matrix is viewed as a transformation of a plane, then the transformation rotates points through an angle equal to the argument of the complex number and scales by a factor equal to the complex number's absolute value. The conjugate of the complex number z corresponds to the transformation which rotates through the same angle as z but in the opposite direction, and scales in the same manner as z; this can be described by the transpose of the matrix corresponding to z.
If the matrix elements are themselves complex numbers, then the resulting algebra is that of the quaternions. In this way, the matrix representation can be seen as a way of expressing the Cayley-Dickson construction of algebras.
Some properties
Real vector space
C is a two-dimensional real vector space. Unlike the reals, complex numbers cannot be ordered in any way that is compatible with its arithmetic operations: C cannot be turned into an ordered field.
Solutions of polynomial equations
A root of the polynomial p is a complex number z such that p(z) = 0. A most striking result is that all polynomials of degree n with real or complex coefficients have exactly n complex roots (counting multiple roots according to their multiplicity). This is known as the Fundamental Theorem of Algebra, and shows that the complex numbers are an algebraically closed field.
Indeed, the complex number field is the algebraic closure of the real number field. It can be identified as the quotient ring of the polynomial ring R[X] by the ideal generated by the polynomial X2 + 1:
- <math> \mathbb{C} = \mathbb{R}[ X ] / ( X^2 + 1). \,<math>
This is indeed a field because X2 + 1 is irreducible. The image of X in this quotient ring becomes the imaginary unit i.
Algebraic characterization
The field C is (up to field isomorphism) characterized by the following three facts:
- its characteristic is 0
- its transcendence degree over the prime field is the cardinality of the continuum
- it is algebraically closed
Consequently, C contains many proper subfields which are isomorphic to C. Another consequence of this characterization is that the Galois group of C over the rational numbers is enormous, with cardinality equal to the power set of the continuum.
Characterization as a topological field
As noted above, the algebraic characterization of C fails to capture some of its most important properties. These properties, which underpin the foundations of complex analysis, arise from the topology of C. The following properties characterize C as a topological field:
- C is a field.
- C contains a subset P of nonzero elements satisfying:
- P is closed under addition, multiplication and taking inverses.
- If x and y are distinct elements of P, then either x-y or y-x is in P
- If S is any nonempty subset of P, then S+P=x+P for some x in C.
- C has a nontrivial involutive automorphism x->x*, fixing P and such that xx* is in P for any nonzero x in C.
Given these properties, one can then define a topology on C by taking the sets
- <math>B(x,p) = \{y | p - (y-x)(y-x)^*\in P\}<math>
as a base, where x ranges over C, and p ranges over P.
To see that these properties characterize C as a topological field, one notes that P ∪ {0} ∪ -P is an ordered Dedekind-complete field and thus can be identified with the real numbers R by a unique field isomorphism. The last property is easily seen to imply that the Galois group over the real numbers is of order two, completing the characterization.
Pontryagin has shown that the only connected locally compact topological fields are R and C. This gives another characterization of C as a topological field, since C can be distinguished from R by noting the nonzero complex numbers are connected whereas the nonzero real numbers are not.
Complex analysis
The study of functions of a complex variable is known as complex analysis and has enormous practical use in applied mathematics as well as in other branches of mathematics. Often, the most natural proofs for statements in real analysis or even number theory employ techniques from complex analysis (see prime number theorem for an example). Unlike real functions which are commonly represented as two dimensional graphs, complex functions have four dimensional graphs and may usefully be illustrated by color coding a three dimensional graph to suggest four dimensions, or by animating the complex function's dynamic transformation of the complex plane.
Applications
Control theory
In control theory, systems are often transformed from the time domain to the frequency domain using the Laplace transform. The system's poles and zeros are then analyzed in the complex plane. The root locus, Nyquist plot, and Nichols plot techniques all make use of the complex plane.
In the root locus method, it is especially important whether the poles and zeros are in the left or right half planes, i.e. have real part greater than or less than zero. If a system has poles that are
- in the right half plane, it will be unstable,
- all in the left half plane, it will be stable,
- on the imaginary axis, it will be marginally stable.
If a system has zeros in the right half plane, it is a nonminimum phase system.
Signal analysis
Complex numbers are used in signal analysis and other fields as a convenient description for periodically varying signals. The absolute value |z| is interpreted as the amplitude and the argument arg(z) as the phase of a sine wave of given frequency.
If Fourier analysis is employed to write a given real-valued signal as a sum of periodic functions, these periodic functions are often written as the real part of complex valued functions of the form
- <math> f ( t ) = z e^{\mathrm{i}\omega t} \,<math>
where ω represents the angular frequency and the complex number z encodes the phase and amplitude as explained above.
In electrical engineering, this is done for varying voltages and currents. The treatment of resistors, capacitors and inductors can then be unified by introducing imaginary frequency-dependent resistances for the latter two and combining all three in a single complex number called the impedance. (Electrical engineers and some physicists use the letter j for the imaginary unit since i is typically reserved for varying currents and indexes and may come into conflict with i. The alternative letter j is also used frequently for indexes and other purposes. See also section Discussion.)
Improper integrals
In applied fields, the use of complex analysis is often used to compute certain real-valued improper integrals, by means of complex-valued functions. Several methods exist to do this, see methods of contour integration.
Quantum mechanics
The complex number field is also of utmost importance in quantum mechanics since the underlying theory is built on (infinite dimensional) Hilbert spaces over C.
Relativity
In special and general relativity, some formulas for the metric on spacetime become simpler if one takes the time variable to be imaginary.
Applied mathematics
In differential equations, it is common to first find all complex roots r of the characteristic equation of a linear differential equation and then attempt to solve the system in terms of base functions of the form f(t) = ert.
Fluid dynamics
In fluid dynamics, complex functions are used to describe potential flow in 2d.
Fractals
Certain fractals are plotted in the complex plane e.g. Mandelbrot set and Julia set.
History
The earliest fleeting reference to square roots of negative numbers occurred in the work of the Greek mathematician and inventor Heron of Alexandria in the 1st century AD, when he considered the volume of an impossible frustum of a pyramid. They became more prominent when in the 16th century closed formulas for the roots of third and fourth degree polynomials were discovered by Italian mathematicians (see Niccolo Fontana Tartaglia, Gerolamo Cardano). It was soon realized that these formulas, even if one was only interested in real solutions, sometimes required the manipulation of square roots of negative numbers. This was doubly unsettling since not even negative numbers were considered to be on firm ground at the time. The term "imaginary" for these quantities was coined by René Descartes in the 17th century and was meant to be derogatory. (See imaginary number for a discussion of the "reality" of complex numbers.) The 18th century saw the labors of Abraham de Moivre and Leonhard Euler. To De Moivre is due (1730) the well-known formula which bears his name, de Moivre's formula:
- <math>(\cos \theta + i\sin \theta)^{n} = \cos n \theta + i\sin n \theta \,<math>
and to Euler (1748) Euler's formula of complex analysis:
- <math>\cos \theta + i\sin \theta = e ^{i\theta }. \,<math>
The existence of complex numbers was not completely accepted until the geometrical interpretation (see below) had been described by Caspar Wessel in 1799; it was rediscovered several years later and popularized by Carl Friedrich Gauss, and as a result the theory of complex numbers received a notable expansion. The idea of the graphic representation of complex numbers had appeared, however, as early as 1685, in Wallis's De Algebra tractatus.
Wessel's memoir appeared in the Proceedings of the Copenhagen Academy for 1799, and is exceedingly clear and complete, even in comparison with modern works. He also considers the sphere, and gives a quaternion theory from which he develops a complete spherical trigonometry. In 1804 the Abbé Buée independently came upon the same idea which Wallis had suggested, that <math>\pm\sqrt{-1}<math> should represent a unit line, and its negative, perpendicular to the real axis. Buée's paper was not published until 1806, in which year Jean-Robert Argand also issued a pamphlet on the same subject. It is to Argand's essay that the scientific foundation for the graphic representation of complex numbers is now generally referred. Nevertheless, in 1831 Gauss found the theory quite unknown, and in 1832 published his chief memoir on the subject, thus bringing it prominently before the mathematical world. Mention should also be made of an excellent little treatise by Mourey (1828), in which the foundations for the theory of directional numbers are scientifically laid. The general acceptance of the theory is not a little due to the labors of Augustin Louis Cauchy and Niels Henrik Abel, and especially the latter, who was the first to boldly use complex numbers with a success that is well known.
The common terms used in the theory are chiefly due to the founders. Argand called <math>\cos \phi + i * \sin \phi<math> the direction factor, and <math>r = \sqrt{a^2+b^2}<math> the modulus; Cauchy (1828) called <math>\cos \phi + i *\sin \phi<math> the reduced form (l'expression réduite); Gauss used i for <math>\sqrt{-1}<math>, introduced the term complex number for <math>a+bi<math>, and called <math>a^2+b^2<math> the norm.
The expression direction coefficient, often used for <math>\cos \phi + i* \sin \phi<math>, is due to Hankel (1867), and absolute value, for modulus, is due to Weierstrass.
Following Cauchy and Gauss have come a number of contributors of high rank, of whom the following may be especially mentioned: Kummer (1844), Leopold Kronecker (1845), Scheffler (1845, 1851, 1880), Bellavitis (1835, 1852), Peacock (1845), and De Morgan (1849). Möbius must also be mentioned for his numerous memoirs on the geometric applications of complex numbers, and Dirichlet for the expansion of the theory to include primes, congruences, reciprocity, etc., as in the case of real numbers.
Other types have been studied, besides the familiar <math>a + bi<math>, in which i is the root of <math>x^2 + 1 = 0<math>. Thus Ferdinand Eisenstein has studied the type <math>a + bj<math>, <math>j<math> being a complex root of <math>x^3 - 1 = 0<math>. Similarly, complex types have been derived from <math>x^k - 1 = 0<math> (<math>k<math> prime). This generalization is largely due to Kummer, to whom is also due the theory of ideal numbers, which has recently been simplified by Felix Klein (1893) from the point of view of geometry. A further complex theory is due to Evariste Galois, the basis being the imaginary roots of an irreducible congruence,
- <math>F(x) \equiv 0<math> (mod <math>p<math>, a prime).
The late writers (from 1884) on the general theory include Weierstrass, Schwarz, Richard Dedekind, Otto Hölder, Berloty, Henri Poincaré, Eduard Study, and Macfarlane.
The formally correct definition using pairs of real numbers was given in the 19th century.
See also
- Riemann sphere (extended complex plane)
- Complex geometry
- De Moivre's formula
- Euler's identity
- Hypercomplex number
- Leonhard Euler
- Local field
- Phasor (physics)
- Phasor (electronics)
- Quaternions
- Split-complex number
Further reading
- An Imaginary Tale, by Paul J. Nahin; Princeton University Press; ISBN 0691027951 (hardcover, 1998). A gentle introduction to the history of complex numbers and the beginnings of complex analysis.
External links
- Complex numbers at Wikibooks
- John and Betty's Journey Through Complex Numbers (http://mathforum.org/johnandbetty/)
- Complex Number from MathWorld (http://mathworld.wolfram.com/ComplexNumber.html)
- SOS Math - Complex Variables (http://www.sosmath.com/complex/complex.html)
- Windows calculator that supports complex numbers (http://www.binarythings.com/hidigit/)
|
Topics in mathematics related to quantity | |
| Numbers | Natural numbers | Integers | Rational numbers | Constructible numbers | Algebraic numbers | Computable numbers | Real numbers | Complex numbers | Split-complex numbers | Bicomplex numbers | Hypercomplex numbers | Quaternions | Octonions | Sedenions | Superreal numbers | Hyperreal numbers | Surreal numbers | Nominal numbers | Ordinal numbers | Cardinal numbers | p-adic numbers | Integer sequences | Mathematical constants | Large numbers | Infinity |
|
Topics in mathematics related to space | Edit (http://en.wikipedia.org/w/wiki.phtml?title=Template:Space&action=edit) |
| Geometry | Trigonometry | Non-Euclidean geometry | Fractal geometry | Algebraic geometry | |
| Topology | Metric geometry | Algebraic topology | Differential geometry and topology | |
| Linear algebra | Functional analysis |
da:Komplekse tal de:Komplexe Zahl et:Kompleksarv es:Número complejo eo:Kompleksa Nombro fr:Nombre complexe he:מספר מרוכב ko:복소수 is:Tvinntölur it:Numero complesso lt:Kompleksiniai skaičiai nl:Complex getal ja:複素数 no:Komplekst tall pl:Liczby zespolone pt:Números complexos ru:Комплексное число sl:Kompleksno število fi:Kompleksiluku scn:Nummuru complessu sv:Komplexa tal th:จำนวนเชิงซ้อน tr:Karmaşık sayılar zh:複數 (數學)