Torus

Contents

1 Geometry 2 Topology 3 The n-torus 4 See also 5 External link

Geometry

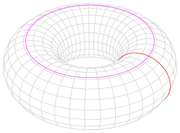
In geometry, a torus (pl. tori) is a doughnut-shaped surface of revolution generated by revolving a circle about an axis coplanar with the circle. The sphere is a special case of the torus obtained when the axis of rotation is a diameter of the circle. If the axis of rotation does not intersect the circle, the torus has a hole in the middle and resembles a ring doughnut, a hula hoop and an inflated tire (U.K. tyre). The other case, when the axis of rotation is a chord of the circle, produces a sort of squashed sphere resembling a round cushion. Torus was the Latin word for a cushion of this shape.

A torus can be defined parametrically by

x(u, v) = (R + r cos v) cos u

y(u, v) = (R + r cos v) sin u

z(u, v) = r sin v

where u, v ∈ [0, 2π], R is the distance from the center of the tube to the center of the torus, and r is the radius of the tube.

The surface area and interior volume of this torus are given by

<math>A = 4\pi^2 Rr<math>

<math>V = 2\pi^2R r^2.<math>

According to a broader definition, the generator of a torus need not be a circle but could also be an ellipse or any other conic section.

Topology

Topologically, a torus is a closed surface defined as product of two circles: S¹ × S¹. One can show that the surface described above, together with the relative topology from R³, is homeomorphic to a topological torus.

The torus can also be described as a quotient of the Euclidean plane under the identifications

(x,y) ~ (x+1,y) ~ (x,y+1)

Or, equivalently, as the quotient of the unit square by pasting the opposite edges together, described as a fundamental polygon <math>ABA^{-1}B^{-1}<math>.

The fundamental group of the torus is just the direct product of the fundamental group of the circle with itself:

<math>\pi_1(\mathbb{T}^2) = \pi_1(S^1) \times \pi_1(S^1) \cong \mathbb{Z} \times \mathbb{Z}<math>

Intuitively speaking, this means that a closed path that circles the torus' "hole" (say, a circle that traces out a particular latitude) and then circles the torus' "body" (say, a circle that traces out a particular longitude) can be deformed to a path that circles the body and then the hole. So, strictly 'latitudinal' and strictly 'longitudinal' paths commute.

The first homology group of the torus is isomorphic to the fundamental group (since the fundamental group is abelian).

The n-torus

One can easily generalize the torus to arbitrary dimensions. An n-torus is defined as a product of n circles:

<math>\mathbb{T}^n = S^1 \times S^1 \times \cdots \times S^1<math>

The torus discussed above is the 2-torus. The 1-torus is just the circle. The 3-torus is rather difficult to visualize. Just as for the 2-torus, the n-torus can be described as a quotient of Rⁿ under integral shifts in any coordinate. That is, the n-torus is Rⁿ modulo the action of the integer lattice Zⁿ (with the action being taken as vector addition). Equivalently, the n-torus is obtained from the n-cube by gluing the opposite faces together.

An n-torus is an example of an n-dimensional compact manifold. It is also an example of a compact abelian Lie group. This follows from the fact that the unit circle is a compact abelian Lie group (when identified with the unit complex numbers with multiplication). Group multiplication on the torus is then defined by coordinate-wise multiplication.

Toroidal groups play an important part in the theory of compact Lie groups. This is due in part to the fact that in any compact Lie group one can always find a maximal torus; that is, a closed subgroup which is a torus of the largest possible dimension.

The fundamental group of an n-torus is a free abelian group of rank n. The k-th homology group of an n-torus is a free abelian group of rank n choose k. It follows that the Euler characteristic of the n-torus is 0 for all n. The cohomology ring H^•(Tⁿ,Z) can be identified with the exterior algebra over the Z-module Zⁿ whose generators are the duals of the n nontrivial cycles.

See also

• toroid
• sphere
• algebraic torus
• elliptic curve
• period lattice
• surface
• torus (nuclear physics)

External link

• Creation of a torus (http://www.cut-the-knot.org/shortcut.shtml#torus) — an avi movie.bg:Тор

de:Torus eo:Toro fr:Tore io:Toro it:Toro_(geometria) ja:トーラス nl:Torus pl:Torus (matematyka) ru:Тор (геометрическая фигура)