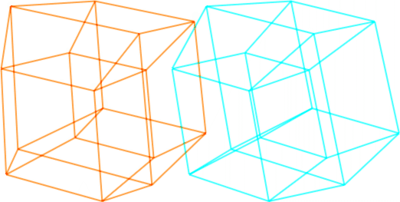
Tesseract
|
|
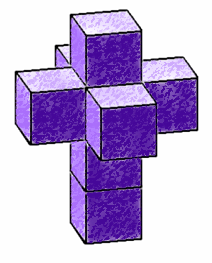 |
The net of a tesseract |
In geometry, the tesseract, or hypercube, is a regular convex polychoron with eight cubical cells. It can be thought of as a 4-dimensional analogue of the cube. Roughly speaking, the tesseract is to the cube as the cube is to the square.
Generalizations of the cube to dimensions greater than three are called hypercubes or measure polytopes. This article focuses on the 4D hypercube, the tesseract.
| Contents [hide] |
Geometry
In a square, each vertex has two perpendicular edges incident to it, while a cube has three. A tesseract has four. Canonical coordinates for the vertices of a tesseract centered at the origin are (±1, ±1, ±1, ±1), while the interior of the same consists of all points (x0, x1, x2, x3) with -1 < xi < 1. This structure is not easily imagined but it is possible to project tesseracts into three or two dimensional spaces. Furthermore, projections on the 2D-plane become more instructive by rearranging the positions of the projected vertices. In this fashion, one can obtain pictures that no longer reflect the spatial relationships within the tesseract, but which nicely illustrate the connection structure of the vertices. The following examples are provided:
| Missing image Hypercube_cubes.png | Missing image Hypercube_star.png | Missing image Hypercube_order.png |
The first illustration shows how a tesseract is in principle obtained by combining two cubes. The scheme is similar to the construction of a cube from two squares: juxtapose two copies of the lower dimensional cube and connect the corresponding vertices. The second picture accounts for the fact that each edge of a tesseract is of the same length. This picture also enables the human brain to find a multitude of cubes that are nicely interconnected. The third diagram finally orders the vertices of the tesseract with respect to the distance along the edges, with respect to the bottom point. This view is of interest when using tesseracts as the basis for a network topology to link multiple processors in parallel computing: the distance between two nodes is at most 4 and there are many different paths to allow weight balancing.
A tesseract is bound by eight hyperplanes. Each pair of non-parallel hyperplanes intersects to form 24 square faces in a tesseract. Three cubes and three squares intersect at each edge. There are four cubes, six squares, and four edges meeting at every vertex. The vertex figure of the tesseract is a regular tetrahedron. Thus the tesseract is given Schläfli symbol {4,3,3}. All in all, it consists of 8 cubes, 24 squares, 32 edges, and 16 vertices. The dual polytope of the tesseract is called the hexadecachoron, or 16-cell, with Schläfli symbol {3,3,4}.
The square, cube, and tesseract are all examples of measure polytopes in their respective dimensions.
Tesseracts are also bipartite graphs, just as a path, rectangle, cube and tree are.
Hypercubes in science fiction
Robert Heinlein mentioned hypercubes in at least two of his science-fiction stories. ...And He Built a Crooked House (1940) described a house built as a net (i.e. an unfolding of the cells into three-dimensional space) of a tesseract. It collapsed, becoming a real hyperdimensional tesseract.
Glory Road (1963) included the foldbox, a hyperdimensional packing case that was bigger inside than outside. It is unclear if Glory Road was influenced by the debut of the science fiction television series Doctor Who on the BBC that same year. In Doctor Who, the main character pilots a time machine called a TARDIS, which is built with technology which makes it "dimensionally transcendental", that is, bigger inside than out.
In addition, a reference can be found in The Number of the Beast (1980), wherein the Burroughs continua device uses the hypercube principle to travel interdimensional universes to the incredible number of the beast.
A hypercube is also used as the main deus ex machina of Robert J. Sawyer's book Factoring Humanity.
The tesseract is mentioned in the children's fantasy novel A Wrinkle In Time, by Madeleine L'Engle, as a way of introducing the concept of higher dimensions, but the treatment is extremely vague. In that book she uses the tesseract as a portal, a doorway which you can pass through and emerge far away from the starting point, as if the two distant points were brought together at one intersection (at the tesseract doorway) by the folding of space, enabling near-instantaneous transportation.
In Alex Garland's 1998 novel "The Tesseract", the author uses the term to mean the three-dimensional net of the four-dimensional hypercube rather than the hypercube itself. It is a metaphor for the characters' inability to understand the causes behind the events which shape their lives: they can only visualize the superficial world they inhabit.
The movie Cube 2: Hypercube focuses on eight strangers trapped inside a "hypercube", or a net of connected hypercubes.
Hypercubes and all kinds of multi-dimensional space and structures star prominently in many books by Rudy Rucker.
The DC Comics crossover DC One Million showed a future Earth in which cities occupied extradimensional areas called tesseracts, leaving the planet's surface unspoiled. Similar technology was used for Superman's current Fortress of Solitude, and was used as storage space in the headquarters of the original incarnation (pre-Zero Hour) of the Legion of Super-Heroes.
The television series Andromeda makes use of tesseract generators as a plot device. These are primarily intended to manipulate space (also referred to as phase shifting) but often cause problems with time as well.
Another TV series, Strange Days at Blake Holsey High (also known as Black Hole High), features an episode (The Tesseract) where Lucas gets trapped in a tesseract. Lucas calls someone he thinks will help (Corrine), but she gets sucked into the tesseract. Eventually, the school folds up in time as well as space. With the help of a "disappeared" teacher, Lucas unfolds the tesseract. The episode gave a good description of a tesseract (see [1] (http://www.tvtome.com/StrangeDaysatBlakeHolseyHigh/)).
In the Nickelodeon TV show Jimmy Neutron, Jimmy doesn't want to eat his corn. So he casually puts it into his Four Dimensional Hypercube that he made himself.
Hypercubes in art
The painting "Crucifixion (Corpus Hypercubus)", by Salvador Dalí, 1954, depicts the crucified Jesus upon the net of a hypercube. It is featured at the Metropolitan Museum of Art. See [2] (http://www.hammondgallery.co.uk/view_pic.php3?aid=44&pid=578)
See also
External links
- HyperSolids (http://uoregon.edu/~koch/hypersolids/hypersolids.html) is an open source program for the Apple Macintosh (Mac OS X and higher) which generates the five regular solids of three-dimensional space and the six regular hypersolids of four-dimensional space.
- an illustration (http://www.geocities.com/hjsmithh/WireFrame4/tesseract.html) (requires Java)
- Hypercube 98 (http://www.mathcs.sjsu.edu/faculty/rucker/hypercube.htm) A Windows program that displays animated hypercubes, by Rudy Rucker
- Flash Demonstration and Interactive Hypercube (http://4d.shadowpuppet.net) (requires Flash)
- ken perlin's home page (http://mrl.nyu.edu/~perlin/demox/Hyper.html) A way to visualize hypercubes, by Ken Perlin
- Magic Cube 4D (http://www.superliminal.com/cube/cube.htm) - Tesseract version of Rubik's Cube
- Cut The Knot!: The Tesseract: An interactive column using Java applets (http://www.cut-the-knot.org/ctk/Tesseract.shtml) by Alex Bogomolny, March 2000
Template:4D regular polytopesde:Tesserakt es:Tesseract pl:Tesserakt ru:Тессеракт sl:hiperkocka