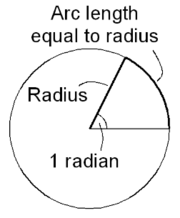
Radian
|
|
In mathematics and physics, the radian is a unit of angle measure. It is the SI derived unit of angle. It is defined as the angle subtended at the center of a circle by an arc of circumference equal in length to the radius of the circle. Angle measures in radians are often given without any explicit unit. When a unit is given, sometimes the abbreviation rad is used, sometimes the symbol c (for "circular").
There are 2π (about 6.283185) radians in a complete circle, so:
2π rad = 360°
1 rad = 360/(2π)° = 180/π° (approximately 57.29578°).
or:
- <math>360^\circ=2\pi\mbox{rad}<math>
- <math>1^\circ=\frac{2\pi}{360}\mbox{rad}=\frac{\pi}{180}\mbox{rad}<math>
In calculus, angles must be represented in radians in trigonometric functions, to make identities and results as simple and natural as possible. For example, the use of radians leads to the simple identity
- <math>\lim_{h\rightarrow 0}\frac{\sin h}{h}=1<math>,
which is the basis of many other elegant identities in mathematics, including
- <math>\frac{d \sin x}{dx} = \cos x<math>.
The radian was formerly an SI supplementary unit, but this category was abolished from the SI system in 1995.
For measuring solid angles, see steradian.
Dimensional analysis
Although the radian is a unit of measure, anything measured in radians is dimensionless. This can be seen easily in that the ratio of an arc's length to its radius is the angle of the arc, measured in radians; yet the quotient of two distances is dimensionless.
Another way to see the dimensionlessness of the radian is in the Taylor series for the trigonometric function <math>\sin(x)<math>:
- <math>\sin(x) = x - \frac{x^3}{3!} + \cdots<math>
If <math>x<math> had units, then the sum would be meaningless; the linear term <math>x<math> can not be added to the cubic term <math>x^3/3!<math>, etc. Therefore, <math>x<math> must be dimensionless.
See also
da:Radian de:Bogenmaß et:Radiaan es:Radián fr:Radian gl:Radián it:Radiante he:רדיאן nl:Radiaal ja:ラジアン ko:라디안 pl:Radian pt:Radiano ru:Радиан sl:Radian fi:Radiaani sr:Радијан sv:Radian uk:Радіан