Phase (waves)

The phase of a wave relates the position of a feature, typically a peak or a trough of the waveform, to that same feature in another part of the waveform (or, which amounts to the same, on a second waveform). The phase may be measured as a time, distance, a fraction of the wavelength, or as an angle.

A phase shift is simply a difference or change in phase.

Mathematically the phase is the argument of a function:
<math>f=\sin (\omega * t + x)\,<math>

where <math>(\omega * t +x )\,<math> represents the argument. To get a grasp of it, consider the two waves A and B in this diagram:

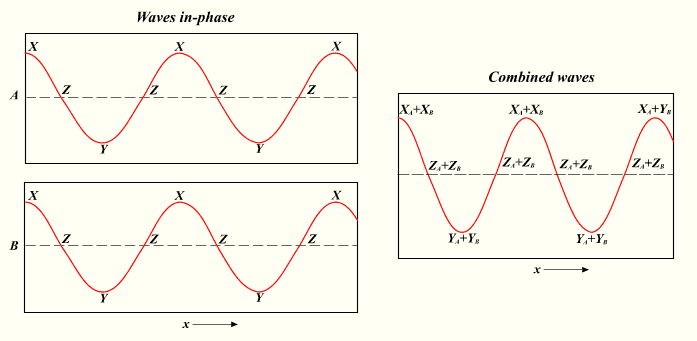
Both A and B have the same amplitude and the same wavelength.

It is apparent that the positions of the peaks (X), troughs (Y) and zero-crossing points (Z) of both waves all coincide. The phase difference of the waves is thus zero, or, the waves are said to be in phase.

If the two in-phase waves A and B are added together (for instance, if they are two light waves shining on the same spot), the result will be a third wave of the same wavelength as A and B, but with twice the amplitude. This is known as constructive interference.

Now consider waves A and C:

Missing image
Outphase.png
Image:outphase.png

A and C are also of the same amplitude and wavelength. However, it can be seen that although the zero-crossing points (Z) are coincident between A and C, the positions of the peaks and troughs are reversed, that is an X on A becomes a Y on C, and vice versa. In this case, the two waves are said to be out of phase or in antiphase, or the phase difference of the two waves is π radians, or half the wavelength (λ/2).

If waves A and C are added, the result is a wave of zero amplitude. This is called destructive interference.

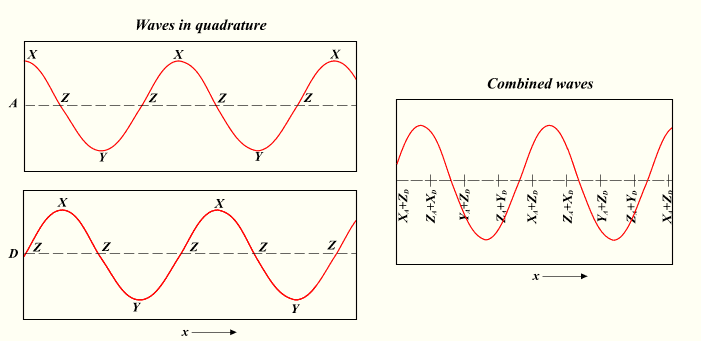
Also consider waves A and D:

In this situation, a peak (X) on wave A becomes a zero-crossing point (Z) on D, a zero-point becomes a peak, and so on. The waves A and D can be said to be in quadrature, or exactly π/2, or λ/4 out of phase.

In nature waveforms are often encountered as sine waves, because of the ubiquitous harmonic motion in physics. In this case the wave amplitude φ is given as a function of a variable, say x, by φ(x) = A sin(α x + φ₀). In such an expression the constant φ₀ is called the phase of the sine (the other constant A is the amplitude). If we plot this function, varying the value of φ₀ results in translating the curve, i.e., to take a new relative "observational point". As it is easier to work with exponentials, the expression would more profitably be written φ(x) = A exp(i(αx + φ₀)), with i the square root of −1. There we can factor φ₀ and consequently exp(iφ₀) is called a pure phase since it contains only phase information and multiplying a function by such a complex exponential changes its phase only.

Coherence is the quality of a wave to display well defined phase relationship in different regions of its domain of definition.

In physics, quantum mechanics ascribes waves to physical objects. The wave function is complex and since its square modulus is associated to probability of observing the object, the complex character of the wave function is associated to the phase. Since the complex algebra is responsible for the striking interference effect of quantum mechanics, phase of particles is therefore ultimately related to their quantum behavior.

It is common to speak of inverting the polarity of a wave as "flipping the phase" or "shifting the phase by 180 degrees". These are not completely equivalent, though, since a 180 degree phase shift of all signal frequencies would also delay the signal. Inverting the signal is instantaneous.

See also

• Phase, for other meanings of the word phase.
• Absolute phaseeo:Fazo

nl:Fase (golf) ja:位相 zh:相位