Octahedron
|
|
| Octahedron | |
|---|---|
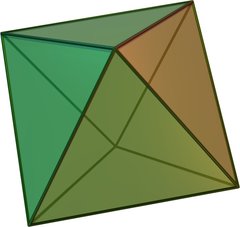 Click on picture for large version. Click here for spinning version. | |
| Type | Platonic |
| Face polygon | triangle |
| Faces | 8 |
| Edges | 12 |
| Vertices | 6 |
| Faces per vertex | 4 |
| Vertices per face | 3 |
| Symmetry group | octahedral (Oh) |
| Dual polyhedron | cube |
| Properties | regular, convex |
An octahedron (plural: octahedra) is a polyhedron with eight faces. A regular octahedron is a Platonic solid composed of eight faces each of which is an equilateral triangle four of which meet at each vertex. The regular octahedron is a special kind of triangular antiprism and of square bipyramid, and is dual to the cube. Canonical coordinates for the vertices of an octahedron centered at the origin are (±1,0,0), (0,±1,0), (0,0,±1).
Missing image
Octahedron_flat.png
image:octahedron flat.png
The area A and the volume V of a regular octahedron of edge length a are:
- <math>A=2\sqrt{3}a^2<math>
- <math>V=\begin{matrix}{1\over3}\end{matrix}\sqrt{2}a^3<math>
The interior of the compound of two dual tetrahedra is an octahedron, and this compound, called the stella octangula, is its first and only stellation. The vertices of the octahedron lie at the midpoints of the faces of the tetrahedron, and in this sense it relates to the tetrahedron in the same way that the cuboctahedron and icosidodecahedron relate to the other Platonic solids. One can also divide the edges of an octahedron in the ratio of the golden mean to define the vertices of an icosahedron. There are five octahedra that define any given icosahedron in this fashion, and together they define a regular compound.
Octahedra and tetrahedra can be mixed together to form a vertex, edge, and face-uniform tiling of space. This is the only such tiling save the regular tessellation of cubes, and is one of the 28 Andreini tessellations. Another is a tessellation of octahedra and cuboctahedra.
Using the standard nomenclature for Johnson solids, an octahedron would be called a square bipyramid.
Especially in roleplaying, this solid is known as a d8, one of the more common Polyhedral dice.
If each edge of an octahedron is replaced by a one ohm resistor, the resistance between opposite vertices is 0.5 ohms, and that between adjacent vertices 5/12 ohms.
See also
External links
- Octahedron (http://mathworld.wolfram.com/Octahedron.html) - Mathworld.com
- The Uniform Polyhedra (http://www.mathconsult.ch/showroom/unipoly/)
- Virtual Reality Polyhedra (http://www.georgehart.com/virtual-polyhedra/vp.html) The Encyclopedia of Polyhedra
- Paper Models of Polyhedra (http://www.korthalsaltes.com/) Many linksca:Octàedre
da:Oktaeder de:Oktaeder es:Octaedro fr:Octaèdre it:Ottaedro ja:正八面体 nl:Octaëder pl:Ośmiościan foremny pt:Icosaedro sv:Oktaeder
