Paradox
|
|
- For other meanings of "Paradox", see Paradox (disambiguation).
Boyle'sSelfFlowingFlask.png
A paradox is an apparently true statement or group of statements that seems to lead to a contradiction or to a situation that defies intuition. Typically, either the statements in question do not really imply the contradiction; or the puzzling result is not really a contradiction; or the premises themselves are not all really true (or, cannot all be true together). The recognition of ambiguities, equivocations, and unstated assumptions underlying known paradoxes has often led to significant advances in science, philosophy and mathematics.
The word paradox is often used interchangeably with contradiction; but where a contradiction by definition cannot be true, many paradoxes do allow of resolution, though many remain unresolved or only contentiously resolved (such as Curry's paradox). Still more casually, the term is sometimes used for situations that are merely surprising (albeit in a distinctly "logical" manner) such as the Birthday Paradox. This is also the usage in economics, where a paradox is an unintuitive outcome of economic theory.
The etymology of paradox can be traced back to the early Renaissance. Early forms of the word appeared in the late Latin paradoxum and the related Greek paradoxon. The word is composed of the preposition para which means "by way of", or "according to" conjoined to the noun stem doxa, meaning "what is received". Compare orthodox (literally, "straight teaching") and heterodox (literally, "different teaching"). The liar paradox and other paradoxes were studied in medieval times under the heading insolubilia.
Common themes in paradoxes include direct and indirect self-reference, infinity, circular definitions, and confusion of levels of reasoning. Paradoxes which are not based on a hidden error generally happen at the fringes of context or language, and require extending the context (or language) to lose their paradox quality.
In moral philosophy, paradox plays a particularly central role in debates on ethics. For instance, an ethical admonition to "love thy neighbor" is not just in contrast with, but in contradiction to an armed neighbor actively trying to kill you: if he or she succeeds, you will not be able to love him or her. But to preemptively attack them or restrain them is not usually understood as very loving. This might be termed an ethical dilemma. Another example is the conflict between an injunction not to steal and one to care for a family that you cannot afford to feed without stolen money.
| Contents |
Types of paradoxes
W. V. Quine (1962) distinguished three classes of paradox:
- A veridical paradox produces a result that appears absurd but is demonstrated to be true nevertheless. Thus, the paradox of Frederic's birthday in The Pirates of Penzance establishes the surprising fact that a person may be more than N years old on his Nth birthday. Likewise, Arrow's impossibility theorem involves behavior of voting systems that is surprising but all too true.
- A falsidical paradox establishes a result that not only appears false but actually is false; there is a fallacy in the supposed demonstration. The various invalid proofs (e.g. that 1 = 2) are classic examples, generally relying on a hidden division by zero. Another example would be the Horse paradox.
- A paradox which is in neither class may be an antinomy, which reaches a self-contradictory result by properly applying accepted ways of reasoning. For example, the Grelling-Nelson paradox points out genuine problems in our understanding of the ideas of truth and description.
List of paradoxes
Quine's classification, of course, is useful only once a paradox has a clear resolution. That question is treated on the page for each individual paradox; the following are grouped thematically.
Logical (except mathematical)
- Paradox of entailment: Inconsistent premises always make an argument valid.
- Raven paradox (or Hempel's Ravens): Observing a red apple increases the likelihood of all ravens being black.
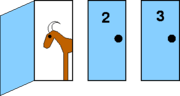
- Horse paradox: All horses are the same colour.
- Unexpected hanging paradox: The day of the hanging will be a surprise, so it can't happen at all, so it will be a surprise.
- Barber paradox: The barber who shaves all men who don't shave themselves, and no-one else. (A close relative of Russell's paradox.)
- Richard's paradox: We appear to be able to use simple English to define a decimal expansion in a way which is self-contradictory.
Semantic paradoxes
These form a well-known (and well-studied) class having in common that any permissible assignment of semantic value (truth, reference) to an expression immediately implies the assignment of a different value.
- Berry paradox: What is "The first number not nameable in under ten words"? (And hasn't it just been named in nine?)
- Curry's paradox: "If I'm not mistaken, the world will end in a week."
- Epimenides paradox: A Cretan says "All Cretans are liars".
- Grelling-Nelson paradox: Is the word "heterological", meaning "not applicable to itself," a heterological word?
- Liar paradox: "This sentence is false."
- Quine's liar paradox: "Yields a falsehood when appended to its own quotation."
- Russell's paradox: Does the set of all those sets that do not contain themselves contain itself?
- The Y combinator in the lambda calculus and combinatory logic has been called the paradoxical combinator since it is related to the self-referential antinomies.
Vagueness
- Ship of Theseus/George Washington's axe: When every component of the ship has been replaced at least once, is it still the same ship?
- Sorites paradox: At what point does a heap stop being a heap as I take away grains of sand? Alternately, at what point does someone become bald?
Mathematical and statistical
- Apportionment paradox: Some systems of apportioning representation can have unintuitive results
- Will Rogers phenomenon: the mathematical concept of an average, whether defined as the mean or median, leads to apparently paradoxical results - for example, it is possible that moving an entry from Wikipedia to Wiktionary would increase the average entry length on both sites
- Berkson's paradox
- Bertrand's paradox (probability): Different common-sense definitions of randomness give quite different results.
- Birthday paradox: What is the chance that two people in a room have the same birthday?
- Borel's paradox: Conditional probability density functions are not invariant under coordinate transformations.
- Elevator paradox: Elevators can seem to be mostly going in one direction, as if they were being manufactured in the middle of the building and being disassembled on the roof and basement.
- Hodgson's paradox: the ratio of two Gaussian random variables has neither mean nor variance.
- Richard's paradox: A complete list of definitions of real numbers doesn't exist.
- Monty Hall problem: An unintuitive consequence of conditional probability.
- Simpson's paradox: An association in sub-populations may be reversed in the population. It appears that two sets of data separately support a certain hypothesis, but, when considered together, they support the opposite hypothesis.
- Sleeping beauty paradox: One half or one third? news://rec.puzzles cannot agree on a probability.
- Statistical paradox: It is quite possible to draw wrong conclusions from correlation. For example, towns with a larger number of churches generally have a higher crime rate — because both result from higher population. A professional organization once found that economists with a Ph.D. actually had a lower average salary than those with a BS — but this was found to be due to the fact that those with a Ph.D. worked in academia, where salaries are generally lower. This is also called a spurious relationship.
- Low birth weight paradox: Low birth weight babies have a higher mortality rate, babies of smoking mothers have lower average birth weight, babies of smoking mothers have a higher mortality rate, but low birth weight babies of smoking mothers have a lower mortality rate than other low birth weight babies.
- Two-envelope paradox: Given two envelopes, one of which contains twice as much money as the other, the benefit seems always to lie in switching from one to the other.
Infinity
- Burali-Forti paradox: If the ordinal numbers formed a set, it would be an ordinal number which is smaller than itself.
- Galileo's paradox: Though most numbers are not squares, there are no more numbers than squares. (See also Cantor, Diagonal Argument)
- Hilbert's paradox of the Grand Hotel: If a hotel with infinitely many rooms is full, it can still take in more guests.
- Monty Hell problem: Positive daily profits yield zero assets in the (infinite) limit.
- Skolem's paradox: Countably infinite models of set theory contain uncountably infinite sets.
Geometry and topology
- Banach-Tarski paradox: Cut a ball into 5 pieces, re-assemble the pieces to get two balls, both of equal size to the first.
- Gabriel's Horn or Torricelli's trumpet: A simple object with finite volume but infinite surface area. Also, the Mandelbrot set and various other fractals have finite area, but infinite perimeter.
- Hausdorff paradox: There exists a countable subset C of the sphere S such that S\C is equidecomposable with two copies of itself.
- Smale's paradox: A sphere can, topologically, be turned inside out.
Psychological, action, and practical reason
- Abilene paradox: People can make decisions based not on what they actually want to do, but on what they think that other people want to do, with the result that everybody decides to do something that nobody really wants to do, but only what they thought that everybody else wanted to do.
- Buridan's ass: How can a rational choice be made between two outcomes of equal value?
- Control paradox: Man can never be free of control, for to be free of control is to be controlled by oneself.
- Paradox of hedonism: When one pursues happiness itself, one is miserable; but, when one pursues something else, one achieves happiness.
- Newcomb's paradox: How do you play a game against an omniscient opponent?
- Kavka's toxin puzzle: Can one intend to drink the nondeadly toxin, if the intention is the only thing needed to get the reward?
- Socratic Paradox: One can never knowingly choose the lesser good or willingly do the wrong thing.
- Cursed Friend: If one is cursed into hurting one’s friends by just being with them, can one still be a friend and seek the other's company?
- Paradox of Denial: How can one accept oneself as one is, if one is not the way one thinks one should be? Yet, how does one effectively change oneself without accepting oneself as one is, and working from that?
- Hypocritical paradox: I hate hypocrites, but one thing I hate even more is intolerant hypocrites.
Physical
Main article: physical paradox
- Braess' paradox: Sometimes adding extra capacity to a network can reduce overall performance.
- Carroll's paradox: the angular momentum of a stick should be zero, but isn't
- D'Alembert's paradox: An inviscid liquid produces no drag.
- Denny's paradox: Surface-dwelling arthropods (such as the water strider) should not be able to propel themselves horizontally.
- Einstein-Podolsky-Rosen paradox: Can far away events influence each other in quantum mechanics?
- Gibbs paradox: In an ideal gas, is entropy an extensive variable?
- The GZK paradox: High-energy cosmic rays have been observed which seem to violate the Greisen-Zatsepin-Kuzmin limit which is a consequence of special relativity.
- Loschmidt's paradox: Why is there an inevitable increase in entropy when the laws of physics are invariant under time reversal? The time reversal symmetry of physical laws appears to allow the second law of thermodynamics to be broken.
- Mpemba paradox: Hot water can under certain conditions freeze faster than cold water, even though it must pass the lower temperature on the way to freezing.
- Olbers' paradox Why is the night sky black if there is an infinity of stars?
- Twin paradox: When the travelling twin returns, he's younger and older than his brother who stayed put.
- Black hole information paradox
- Supplee's paradox: the buoyancy of a relativistic object (such as a bullet) appears to change when the reference frame is changed from one in which the bullet is at rest to one in which the fluid is at rest
Philosophy
- Fermi paradox: If there are many other sentient species in the Universe, then where are they? Shouldn't their presence be obvious?
- Grandfather paradox: You travel back in time and kill your grandfather before he meets your grandmother which precludes your own conception and, therefore, you couldn't go back in time and kill your grandfather.
- Mere addition paradox: Is a large population living barely tolerable lives better than a small happy population?
- Nihilist paradox: If truth does not exist, the statement "truth does not exist" is a truth, thereby proving itself incorrect.
- Omnipotence paradox: Can an omnipotent being create a rock too heavy to lift? Can an irresistible force move an unmovable object?
- Predestination paradox: A man travels back in time and impregnates his great-great-grandmother. The result is a line of offspring and descendants, including the man's parent(s) and the man himself. Therefore, unless he makes the time-travel trip at all, he will never exist.
- Epicurean paradox, or Problem of evil: The existence of evil is incompatible with the existence of an omnipotent and caring God.
- Moore's paradox: "It's raining but I don't believe that it is."
- Zeno's paradoxes: When you reach the turtle's spot, it has already advanced a bit, so you can never catch it.
- Locudox - The opposite of a Paradox: First imagined by Portugese Mathematician Pedro Nunes. Given the human brain can describe a paradox it must be able to contain it, and the state where any given paradox ceases to display its inherent conflicting characteristics is decribed as a Locudox.
Economics
- Abilene paradox: A group of people often has to decide against its own interests.
- Arrow's paradox/Voting paradox/Condorcet paradox: You can't have all the attributes of an ideal voting system at once.
- Bertrand paradox: Two players reaching a state of Nash equilibrium both find themselves with no profits.
- Bird in the bush paradox: Why are people so risk-averse?
- Diamond-water paradox Why is water cheaper than diamonds, when humans need water to survive, not diamonds?
- Edgeworth paradox: with capacity constraints, there may not be an equilibrium.
- Ellsberg paradox: A paradoxical result in experimental decision theory.
- Giffen paradox: Can increasing the price of bread make poor people eat more of it?
- Jevons paradox: Increases in efficiency lead to even larger increases in demand.
- St. Petersburg paradox: People will only offer a modest fee for a reward of infinite value.
References
- Quine, W. V. (1962). "Paradox". Scientific American, April 1962, pp. 84–96.
- Clarke, Michael (2002). Paradoxes from A to Z. London: Routledge.
See also
External links
- Open Directory Project: Paradoxes (http://www.dmoz.org/Society/Philosophy/Philosophy_of_Logic/Paradoxes/)
- Definability paradoxes (http://www.dpmms.cam.ac.uk/~wtg10/richardsparadox.html)
- Insolubles (http://plato.stanford.edu/entries/insolubles) (at the Stanford Encyclopedia of Philosophy (http://plato.stanford.edu))da:Paradoks
de:Paradoxon es:Paradoja eo:Paradokso fi:Paradoksi fr:Paradoxe he:פרדוקס io:Paradoxo ja:パラドックス nl:Paradox pl:Paradoks pt:Paradoxo ru:Парадокс simple:Paradox sv:Paradox zh:悖论
