Twin paradox

The Twin paradox is a thought experiment in special relativity of two twin brothers, one undertaking a long space journey with a very high-speed rocket at almost the speed of light, the other remaining on Earth. When the traveler finally returns to Earth, it is observed that he is younger than the twin who stayed put. Or, as first stated by Albert Einstein (1911):

If we placed a living organism in a box ... one could arrange that the organism, after any arbitrary lengthy flight, could be returned to its original spot in a scarcely altered condition, while corresponding organisms which had remained in their original positions had already long since given way to new generations. For the moving organism the lengthy time of the journey was a mere instant, provided the motion took place with approximately the speed of light. (in Resnick and Halliday, 1992)

This outcome is predicted by Einstein's special theory of relativity. It is due to an experimentally verified phenomenon called time dilation, in which a moving clock is found to experience a reduced amount of proper time as determined by clocks synchronized with a stationary clock. One example is with muons produced in the upper atmosphere being detectable on the ground. Without time dilation, the muons would decay long before reaching the ground. Another experiment confirmed time dilation by comparing the effects of speed on two atomic clocks, one based on earth, the other aboard a supersonic plane. They were out of sync afterwards, with the one on the plane being slightly behind.

The paradox arises if one takes the position of the traveling twin: from his perspective, his brother on Earth is moving away quickly, and eventually comes close again. So the traveler can regard his brother on Earth to be a "moving clock" which should experience time dilation. Special relativity says that all observers are equivalent, and no particular frame of reference is privileged. Hence, the traveling twin, upon return to Earth, would expect to find his brother to be younger than himself, contrary to that brother's expectations. Which twin is correct?

It turns out that the traveling twin's expectation is mistaken: special relativity does not say that all observers are equivalent, only that all observers in inertial frames are equivalent. But the traveling twin jumps frames when he does a U-turn. The twin on Earth rests in the same inertial frame for the whole duration of the flight (no accelerating or decelerating forces apply to him) and he is therefore able to distinguish himself from the traveling twin.

There are not two but three relevant inertial frames: the one in which the stay-at-home twin remains at rest, the one in which the traveling twin is at rest on his outward trip, and the one in which he is at rest on his way home. It is during the acceleration and deceleration of the departure and arrival to Earth and similar accelerations at the U-turn when the traveling twin switches frames. That's when he must adjust the calculated age of the twin at rest. This is a purely artificial effect caused by the change in the definition of simultaneity when changing frames. Here's why.

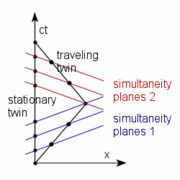
In special relativity there is no concept of absolute present. A present is defined as a set of events that are simultaneous from the point of view of a given observer. The notion of simultaneity depends on the frame of reference, so switching between frames requires an adjustment in the definition of the present. If one imagines a present as a (three-dimensional) simultaneity plane in Minkowski space, then switching frames results in changing the inclination of the plane.

In the spacetime diagram on the right, the first twin's lifeline coincides with the vertical axis (his position is constant in time). On the first leg of the trip, the second twin moves to the right (black sloped line); and on the second leg, back to the left. Blue lines show the planes of simultaneity for the traveling twin during the first leg of the journey; red lines, during the second leg. During the U-turn the plane of simultaneity jumps from blue to red and very quickly sweeps a large segment of the lifeline of the resting twin. Suddenly the resting twin "ages" very fast in the reckoning of the traveling twin.

It is sometimes claimed that the twin paradox cannot be resolved without the use of general relativity, since one of the twins must undergo acceleration during the U-turn. This is false, for two reasons. First, most simply, the acceleration can easily be made to be a negligible part of the trip by making the inertial legs long enough. Second, it is no problem, in principle, to describe the effects of acceleration in special relativity as long as one does so using the laws of physics formulated in an inertial frame of reference — general relativity is only needed to make the laws of physics in the accelerated frame the same as in an inertial frame with a gravitational field. As Hermann Bondi once quipped on this question (French, 1968), "it is obvious that no theory denying the observability of acceleration could survive a car trip on a bumpy road," and special relativity certainly does not deny acceleration.

Alternative resolution of paradox

Missing image
Dts0.gif
Image:dts0.gif

Consider a space ship going from Earth to the nearest star system; 4.45 light years away; at 0.866 c. The above image shows the the ship with its 0.5 length contraction. To an observer on Earth the trip will take 5.14 years, producing a round trip time of 10.28 years.

Missing image
Dtsv.gif
Image:dtsv.gif

However, to the ship's crew the stars and the distance between them will be shortened in the direction of motion to 0.5 of what is observed on Earth, resulting in a travel distance of only 2.23 light years and a corresponding 2.57 year travel time. This produces a round trip time of only 5.14 years. Thus the ship's crew would experience less time than those on Earth.

If one of the astronauts on the ship had a twin that stayed on Earth, he would return home to find his brother about 5 years older than himself.

Now, this takes care of the physical effect of time dilation but it does not reconcile how each twin would observe the other during the trip. The solution to this observational problem can be found in the relativistic Doppler effect. It includes the effects of both time dilation and distance, hence we can calculate just how fast each twin will observe time to flow in the other's reference frame.

• The black line is the Earth's path through space time.
• The dark blue line is the ship's outward path through space time.
• The purple line is its return path through space time.
• The green dots are equally spaced points in time within their respective frame of reference.
• The red lines are the path of light between Earth and the ship during the outward trip.
• Light blue dots indicate arrival point of light.

On the outward trip the relativistic Doppler effect shows each twin would see 1 second pass for the other twin for every 3.7 seconds of his own time. The twin on the ship would see this effect for 2.57 years; as such he would see his twin age 0.69 years. However, since the light showing the ship's arrival would not arrive at Earth for 4.45 years, the twin on Earth would see this effect for 9.59 years, as such he would see his twin age 2.57 years.

• Blue lines are the path of light between Earth and the ship during the return trip.

On the inward trip, the relativistic Doppler effect shows each twin would see 3.7 seconds pass for the other twin for every second of his own time. The twin on the ship would see this effect for 2.57 years; as such he would see his twin age 9.59 years, for a total of 10.28 years. However since the light showing the ships departure would not arrive at Earth for 4.45 years, the twin on Earth would see this effect for only 0.69 years since the ship returns after 10.28 years; as such he would see his twin age 2.57 years, for a total of 5.14 years.

• This diagram shows the light path's for both legs of the flight.
• Note that there is more blue Earth-ship and more red Ship-Earth. That is why the ship's crew sees the Earth age more than they do.

Thus the twin paradox is resolved both in terms of the physical effect of time dilation and the observation of both twins, since both the physical affect and observation agree on how much each twin would age.

References

• A. P. French, Special Relativity (W. W. Norton: New York, 1968).
• Robert Resnick and David Halliday, Basic Concepts in Relativity (Macmillan: New York, 1992).

External links

• Usenet Physics FAQ Twin Paradox (http://www.physics.adelaide.edu.au/~dkoks/Faq/Relativity/SR/TwinParadox/twin_paradox.html)
• 1972 Haefele-Keating experiment (http://hyperphysics.phy-astr.gsu.edu/hbase/relativ/airtim.html#c1)de:Zwillingsparadoxon

fr:Paradoxe des jumeaux it:Paradosso dei gemelli nl:Tweelingenparadox ja:双子のパラドックス pl:Paradoks bliźniąt fi:Kaksosparadoksi zh:双生子佯谬