Precession
|
|
Precession (also called gyroscopic precession) is the phenomenon by which the axis of a spinning object (e.g. a part of a gyroscope) "wobbles" when a torque is applied to it. The phenomenon is commonly seen in a spinning toy top, but all rotating objects can undergo precession. As a spinning object precesses, the tilt of its axis goes around in a circle in the opposite direction that the object is spinning. If the speed of the rotation and the magnitude of the torque are constant the axis will describe a cone, its movement at any instant being at right angles to the direction of the torque. In the case of a toy top, if the axis is not perfectly vertical the torque is applied by the force of gravity trying to tip it over. A rolling wheel will tend to remain upright due to precession. When the wheel tilts to one side, the particles at the top are pushed to one side and the particles at the bottom are pushed the other way. However, since the wheel is rotating, these particles eventually switch places and cancel one another out. Precession or gyroscopic considerations may have a minor effect on bicycle performance at high speed. Precession is also the mechanism behind gyrocompasses.
| Contents |
The physics of precession
Precession is due to the fact that the resultant of the angular velocity of rotation and the angular velocity produced by the torque is an angular velocity about a line which makes an angle with the permanent rotation axis, and this angle lies in a plane at right angles to the plane of the couple producing the torque. The permanent axis must turn towards this line, since the body cannot continue to rotate about any line which is not a principal axis of maximum moment of inertia; that is, the permanent axis turns in a direction at right angles to that in which the torque might be expected to turn it. If the rotating body is symmetrical and its motion unconstrained, and if the torque on the spin axis is at right angles to that axis, the axis of precession will be perpendicular to both the spin axis and torque axis. Under these circumstances the period of precession is given by:
- <math>
T_p = \frac{4\pi^2I_s}{QT_s} <math>
In which Is is the moment of inertia, Ts is the period of spin about the spin axis, and Q is the torque. In general the problem is more complicated than this, however.
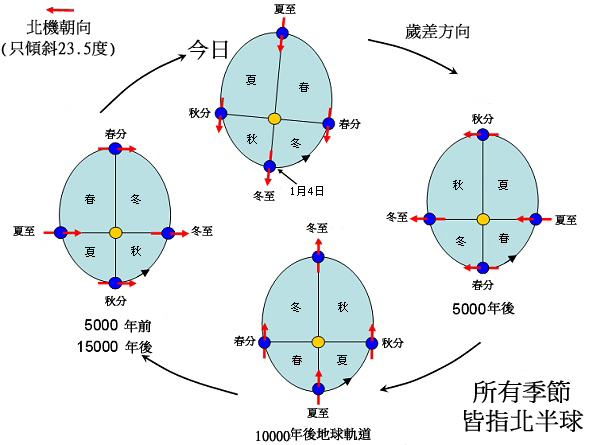
Precession of the equinoxes
The axis of the Earth undergoes precession due to a combination of the Earth's nonspherical shape (it is an oblate spheroid, bulging outward at the equator) and the gravitational tidal forces of the Moon and Sun applying torque as they attempt to pull the equatorial bulge into the plane of the ecliptic. The Earth goes through one complete precession cycle in a period of approximately 25,800 years, during which the positions of stars as measured in the equatorial coordinate system will slowly change; the change is actually due to the change of the coordinates. Over this cycle the Earth's north axial pole moves from where it is now, within 1° of Polaris, in a circle around the ecliptic pole, with an angular radius of about 23.5 degrees. The shift is 1 degree in 180 years (the angle is taken from the observer, not from the center of the circle).
Polaris is not particularly well-suited for marking the north celestial pole, as its visual magnitude, which is variable, hovers around 2.1, fairly far down the list of brightest stars in the sky. On the other hand, in 3000 BC the faint star Thuban in the constellation Draco was the pole star; at magnitude 3.67 it is five times fainter than Polaris; today it is all but invisible in light-polluted urban skies. The brightest star known to have been North Star or to be predictable as taking that role in the future is the brilliant Vega in the constellation Lyra, which will be the pole star around the year 14,000 AD. When viewed looking down onto the Earth from the north, the direction of precession is clockwise. When standing on Earth looking outward, the axis appears to move counter-clockwise across the sky. This sense of precession, against the sense of Earth's own axial rotation, is opposite to the precession of a top on a table. The reason is that the torques imposed on the Earth by the Sun and Moon act in the sense of trying to align its axis normal to the ecliptic, i.e. to stand up more vertically in regards to the ecliptic plane, while the torque on a top spinning on a hard surface acts in the sense of trying to make the top fall over, rather than to stand up straighter.
Missing image
Precession_starchart.jpg
Image:precession starchart.jpg
Polaris is not exactly at the pole; any long-exposure unguided photo will show it having a short trail. It is close enough, though. The south celestial pole precesses too, always remaining exactly opposite the north pole. The south pole is in a particularly bland portion of the sky, and the nominal south pole star is Sigma Octantis, which, while fairly close to the pole, is even weaker than Thuban -- magnitude 5.5, which is barely visible even under a properly dark sky. The precession of the Earth is not entirely regular due to the fact that the Sun and Moon are not in the same plane and move relative to each other, causing the torque they apply to Earth to vary. This varying torque produces a slight irregular motion in the poles called nutation.
Precession of the Earth's axis is a very slow effect, but at the level of accuracy at which astronomers work, it does need to be taken into account. Note that precession has no effect on the inclination ("tilt") of the plane of the Earth's equator (and thus its axis of rotation) on its orbital plane. It is 23.5 degrees and precession does not change that. The inclination of the equator on the ecliptic does change due to gravitational torque, but its period is different (main period about 41000 years).
The following figure illustrates the effects of axial precession on the seasons, relative to perihelion and aphelion. The precession of the equinoxes can cause periodic climate change (see Milankovitch cycles), because the hemisphere that experiences summer at perihelion and winter at aphelion (as the southern hemisphere does presently) is in principle prone to more severe seasons than the opposite hemisphere.
[1] (http://hometown.aol.com/gregbenson/iceage.htm)
Hipparchus first estimated Earth's precession around 130 BC, adding his own observations to those of Babylonian and Chaldean astronomers in the preceding centuries. In particular they measured the distance of the stars like Spica to the Moon and Sun at the time of lunar eclipses, and because he could compute the distance of the Moon and Sun from the equinox at these moments, he noticed that Spica and other stars appeared to have moved over the centuries.
Precession causes the cycle of seasons (tropical year) to be about 20.4 minutes less than the period for the earth to return to the same position with respect to the stars as one year previously (sidereal year). This results in a slow change (one day per 58 calendar years) in the position of the sun with respect to the stars at an equinox. It is significant for calendars and their leap year rules.
Precession of planetary orbits
Perihelion_precession.jpg
The revolution of a planet in its orbit around the Sun is also a form of rotary motion. (In this case, the combined system of Earth and Sun is rotating.) So the axis of a planet's orbital plane will also precess over time.
The major axis of each planet's elliptical orbit also precesses within its orbital plane, in response to perturbations in the form of the changing gravitational forces exerted by other planets. This is called "perihelion precession." Discrepancies between the observed perihelion precession rate of the planet Mercury and that predicted by classical mechanics were prominent among the forms of experimental evidence leading to the acceptance of Einstein's Theory of Relativity, which predicted the anomalies accurately.
The precession of the orbit of the Earth is an important part of the astronomical theory of ice ages. It is generally understood that the gravitational pulls of the sun and the moon cause the precession of the equinoxes; these operate on cycles of 23,000 and 19,000 years.
Precession is also an important consideration in the dynamics of atoms and molecules.
See also
de:Präzession es:Precesión de los equinoccios eo:Precesio fr:Précession ko:세차운동 nl:Precessie nds:Präzession ja:歳差 pl:Precesja vi:Tuế sai zh:岁差