Concentration
|
|
- This page refers to concentration in the chemical sense.
- For the psychological concept of concentration, see attention.
- For the game show of the same name, see Concentration (game show).
Concentration is a very common concept used in chemistry and related fields. It is the measure of how much of a given substance there is mixed with another substance. This can apply to any sort of chemical mixture, but most frequently is used in relation to solutions, where it refers to the amount of solute dissolved in a solvent.
To concentrate a solution, one must add more solute, or reduce the amount of solvent (for instance, by selective evaporation). By contrast, to dilute a solution, one must add more solvent, or reduce the amount of solute.
There exists a concentration at which no further solute will dissolve in a solution. At this point, the solution is said to be saturated. If additional solute is added to a saturated solution, it will not dissolve. Instead, phase separation will occur, leading to either coexisting phases or a suspension. The point of saturation depends on many variables such as ambient temperature and the precise chemical nature of the solvent and solute.
Concentration may be expressed both qualitatively ('informally') or quantitatively ('numerically').
| Contents |
Qualitative Notation
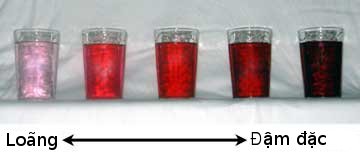
Qualitatively, solutions of relatively low concentration are described using adjectives such as "dilute," or "weak," while solutions of relatively high concentration are described as "concentrated," or "strong." As a rule, the more concentrated a chromatic solution is, the more intensely coloured it is.
Quantitative Notation
Quantitative notation of concentration is far more informative and useful from a scientific point of view. There are a number of different ways to quantitatively express concentration; the most common are listed below.
Note: Many units of concentration require measurement of a substance's volume, which is variable depending on ambient temperature and pressure. Unless otherwise stated, all the following measurements are assumed to be at standard state temperature and pressure (that is, 25 degrees Celsius at 1 atmosphere).
Mass percentage
Mass percentage denotes the mass of a substance in a mixture as a percentage of the mass of the entire mixture. For instance: if a bottle contains 40 grams of ethanol and 60 grams of water, then it contains 40% ethanol by mass. Commercial concentrated aqueous reagents such as acid and bases are often labeled in concentrations of weight percentage with the specific gravity also listed. In older texts and references this is sometimes referred to as weight-weight percentage (abbreviated as w/w).
Mass-Volume percentage
Mass-volume percentage, (sometimes referred to as weight-volume percentage and often abbreviated as % m/v or % w/v) denotes the mass of a substance in a mixture as a percentage of the volume of the entire mixture. Mass-volume percentage is often used for solutions made from solid reagents. It is the mass of the solute in grams multiplied by one hundred divided by the volume of solution in milliliters.
Volume-Volume percentage
Volume-volume percentage or % (v/v) describes the volume of the solute in mL per 100 mL of the resulting solution. This is most useful when a liquid - liquid solution is being prepared. For example, beer is about 5% ethanol by volume. This means every 100 mL beer contains 5 mL ethanol (ethyl alcohol).
Molarity
Molarity (M) denotes the number of moles of a given substance per litre of solution. For instance: 4.0 litres of liquid, containing 2.0 moles of dissolved particles, constitutes a solution of 0.5 M. Such a solution may be described as "0.5 molar." (Working with moles can be highly advantageous, as they enable measurement of the absolute number of particles in a solution, irrespective of their weight and volume. This is often more useful when performing stoichiometric calculations.)
Molality
Molality (m) denotes the number of moles of a given substance per kilogram of solvent. For instance: 2.0 kilograms of solvent, containing 1.0 moles of dissolved particles, constitutes a molality of 0.5 mol/kg. Such a solution may be described as "0.5 molal."
The advantage of molality is that it does not change with the temperature, as it deals with the mass of solvent rather than the volume of solution. Volume increases with increase in temperature resulting in decrease in molarity. Molality of a solution is always constant irrespective of the physical conditions like temperature and pressure.
Molinity
Molinity is a rarely-used term that denotes the number of moles of a given substance per kilogram of solution. For instance: imagine 2.0 kg of solvent, plus 1.0 mol of dissolved particles, weighs a total of 2.5 kg. The molinity of the solution is therefore 1 mol / 2.5 kg = 0.4 mol/kg.
- Note: molarity and molinity are calculated using the volume of the entire solution, but molality is calculated using the mass of solvent only.
- Warning: There may be confusion between above terms, which look and sound very similar; also, the abbreviations 'M' (denoting molarity) and 'm' (denoting molality) can be ambiguous. Special care should be exercised; if there is any risk of confusion, one should fully describe the measure being used.
Normality
Normality is a concept related to molarity, usually applied to acid-base solutions and reactions. For acid-base reactions, the equivalent is the mass of acid or base that can accept or donate exactly one mole of protons (H+ ions). Normality is also used for redox reactions. In this case the equivalent is the quantity of oxidizing or reducing agent that can accept or furnish one mole of electrons.
Whereas molarity measures the number of particles per litre of solution, normality measures the number of equivalents per litre of solution.
In practice, this simply means one multiplies the molarity of a solution by the valence of the ionic solute. A bit more complex for redox reactions.
Note: The normality is always equal to, or greater than the molarity for acid-base reactions. However, for redox reactions the normality is typically equal to or less than the molarity.
Mole Fraction
The mole fraction χ, chi (also called molar fraction) denotes the number of moles of solute as a proportion of the total number of moles in a solution. For instance: 1 mole of solute dissolved in 9 moles of solvent would have a mole fraction of 1/10 or 0.1.
Formal
The formal (F) is yet another measure of concentration similar to molarity. It is used rarely. It is calculated based on the formula weights of chemicals per litre of solution. The difference between formal and molar concentrations is that the formal concentration indicates moles of the original chemical formula in solution, without regard for the species that actually exist in solution. Molar concentration, on the other hand, is the concentration of species in solution.
For example: if one dissolves calcium carbonate (CaCO3) in a litre of water, the compound dissociates into the Ca2+ and CO32- ions. The CO32- further dissociates into HCO3- and H2CO3. There is practically no CaCO3 left in the solution. So, although we have added 1 mol of CaCO3 to the solution, it does not contain 1 M of that substance. (Rather, it contains a molarity based on the other constituents of the solution.) However, one can still say that the solution contains 1 F of CaCO3.
"Parts-per" Notation
The parts-per notation is used for extremely low concentrations. This is often used to denote the relative abundance of trace elements in the Earth's crust, trace elements in forensics or other analyses, or levels of pollutants in the environment.
- Parts per hundred (denoted by '%' and very rarely 'pph') - denotes one particle of a given substance for every 99 other particles. This is the common percent. 1 part in 102.
- Parts per thousand (denoted by '‰' [the per mil symbol], and occasionally 'ppt') denotes one particle of a given substance for every 999 other particles. This is roughly equivalent to one drop of ink in a cup of water, or one second per 17 minutes. 'Parts per thousand' is often used to record the salinity of seawater. 1 part in 103.
- Parts per million ('ppm') denotes one particle of a given substance for every 999,999 other particles. This is roughly equivalent to one drop of ink in a 40 gallon drum of water, or one second per 280 hours. 1 part in 106.
- Parts per billion ('ppb') denotes one particle of a given substance for every 999,999,999 other particles. This is roughly equivalent to one drop of ink in a canal lock full of water, or one second per 32 years. 1 part in 109.
- Parts per trillion ('ppt') denotes one particle of a given substance for every 999,999,999,999 other particles. This is roughly equivalent to one drop of ink in an Olympic-sized swimming pool, or one second every 320 centuries. 1 part in 1012.
- Parts per quadrillion ('ppq'?) denotes one particle of a given substance for every 999,999,999,999,999 other particles. This is roughly equivalent to a drop of ink in a medium-sized lake, or one second every 32,000 millennia. There are no known analytical techniques that can measure with this degree of accuracy; nevertheless, it is still used in some mathematical models of toxicology and epidemiology. 1 part in 1015.
Warning: although 'ppt' is usually used to denote 'parts per trillion', it is also on occasion used to denote 'parts per thousand'. If there is any chance of ambiguity, one should describe the abbreviation in full.
According to the U.S. National Institute of Standards and Technology (NIST) Guide for the Use of the International System of Units (SI), "the language-dependent terms part per million, part per billion, and part per trillion ... are not acceptable for use with the SI to express the values of quantities." [1] (http://physics.nist.gov/Pubs/SP811/sec07.html#7.10.3) which lists examples of alternative expressions.
Notes for clarity:
- The indication given above is that parts per notation refers to numbers of particles (equivalent to moles), whereas in the last column of the chart below it is given by mass (grams per kilogram). Those using the notation need to state their usage to avoid confusion.
- In atmospheric chemistry the parts per notation is commonly expressed with a v following, such as ppmv (or ppvm is some usages), to indicate parts per million by volume. In gases ppmv is equivalent to ppm by particles (Avogadro's law). This works fine for gases, but may have problems with cloud droplets and smoke or other atmospheric particulate matter.
Techniques Used to Determine Concentration
- Spectrophotometry
- Chromatography
- Various titration methods
Table of Concentration Measures
| Measurement | Notation | Generic Formula | Typical Units |
|---|---|---|---|
| Mass Percentage | - | <math>\left ( \frac{grams\ solute \times 100}{grams\ solution} \right )<math> | % |
| Mass-Volume Percentage | - | <math>\left ( \frac{grams\ solute \times 100}{millilitres\ solution} \right )<math> | % though strictly %kg/L |
| Volume-Volume Percentage | - | <math>\left ( \frac{millilitres\ solute \times 100}{millilitres\ solution} \right )<math> | % |
| Molarity | M | <math>\left ( \frac{moles\ solute}{litres\ solution} \right )<math> | mol/L (or M) |
| Molinity | - | <math>\left ( \frac{moles\ solute}{kilograms\ solution} \right )<math> | mol/kg |
| Molality | m | <math>\left ( \frac{moles\ solute}{kilograms\ solvent} \right )<math> | mol/kg (or m) |
| Molar Fraction | χ (chi) | <math>\left ( \frac{moles\ solute}{moles\ solution} \right )<math> | (fraction) |
| Formal | F | <math>\left ( \frac{moles\ undissolved\ solute}{litres\ solution} \right )<math> | mol/L (or F) |
| Normality | N | <math>\left ( \frac{moles\ solute}{litres\ solution} \times valence\ of\ solute \right )<math> | N |
| Parts per hundred | % (or pph) | <math>\left ( \frac{dekagrams\ solute}{kilograms\ solution} \right )<math> | da.g/kg |
| Parts per thousand | ‰ (or ppt*) | <math>\left ( \frac{grams\ solute}{kilograms\ solution} \right )<math> | g/kg |
| Parts per million | ppm | <math>\left ( \frac{milligrams\ solute}{kilograms\ solution} \right )<math> | mg/kg |
| Parts per billion | ppb | <math>\left ( \frac{micrograms\ solute}{kilograms\ solution} \right )<math> | μg/kg |
| Parts per trillion | ppt* | <math>\left ( \frac{nanograms\ solute}{kilograms\ solution} \right )<math> | ng/kg |
| Parts per quadrillion | ppq | <math>\left ( \frac{picograms\ solute}{kilograms\ solution} \right )<math> | pg/kg |
* Although 'ppt' is usually used to denote 'parts per trillion', it is on occasion used for 'parts per thousand'. Beware any ambiguity in usage.
Note (1) : The table above is described in terms of solvents and solutes; however the units given often also apply to other types of mixture.
Note (2) : The use of billion, trillion, quadrillion above follows the short scale usage of these words.de:Stoffkonzentration es:Concentración nl:Concentratie ja:モル濃度 pl:Stężenie sl:Koncentracija fi:Konsentraatio vi:Nồng độ