Menger sponge
|
|
The Menger sponge is a fractal solid. It is also known as the Menger-Sierpinski sponge or, incorrectly, the Sierpinski sponge. It is a three-dimensional extension of the Cantor set and Sierpinski carpet, with Hausdorff dimension (ln 20) / (ln 3) (approx. 2,726833). It was first described by Austrian mathematician Karl Menger in 1926.
| Contents |
Construction
Missing image
Menger_sponge_(Level_1-4).jpg Menger sponge, first four levels of the construction. |
Construction of a Menger sponge can be visualized as follows:
After an infinite number of iterations, a Menger sponge will remain. |
The number of cubes increases by : <math>20^n<math>. Where <math>n<math> is the number of iterations performed on the first cube:
| Iters | Cubes | Sum |
| 0 | 1 | 1 |
| 1 | 20 | 21 |
| 2 | 400 | 421 |
| 3 | 8,000 | 8,421 |
| 4 | 160,000 | 168,421 |
| 5 | 3,200,000 | 3,368,421 |
| 6 | 64,000,000 | 67,368,421 |
At the first level, no iterations are performed, (20 n=0 = 1).
Properties
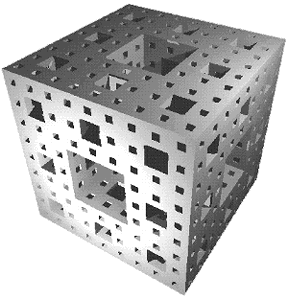
Each face of the Menger sponge is a Sierpinski carpet; furthermore, any intersection of the Menger sponge with a diagonal or medium of the initial cube M0 is a Cantor set. The Menger sponge is a closed set; since it is also bounded, the Heine-Borel theorem yields that it is compact. Furthermore, the Menger sponge is uncountable and has Lebesgue measure 0.
The topological dimension of the Menger sponge is one; indeed, the sponge was first constructed by Menger in 1926 while exploring the concept of topological dimension. Note that the topological dimension of any curve is one; that is, curves are topologically one-dimensional. Menger showed, in the 1926 construction, that the sponge is a universal curve, in that any possible one-dimensional curve is homeomorphic to a subset of the Menger sponge. Note that by curve we mean any object of Lebesgue covering dimension one; this includes trees and graphs with an arbitrary countable number of edges, vertices and closed loops, connected in arbitrary ways.
In a similar way, the Sierpinski gasket is a universal curve for all curves that can be drawn on the two-dimensional plane. The Menger sponge constructed in three dimensions extends this idea to graphs that are not flat, and might be embedded in any number of dimensions. Thus, as a humorous example, any geometry of quantum loop gravity can be embedded in a Menger sponge.
Formal definition
Formally, a Menger sponge can be defined as follows:
- <math>M := \bigcap_{n\in\mathbb{N}} M_n<math>
where M0 is the unit cube and
- <math>M_{n+1} := \left\{\begin{matrix}
(x,y,z)\in\mathbb{R}^3: & \begin{matrix}\exists i,j,k\in\{0,1,2\}: (3x-i,3y-j,3z-k)\in M_n \\ \mbox{and at most one of }i,j,k\mbox{ is equal to 1}\end{matrix} \end{matrix}\right\}<math>
See also
References
- Karl Menger, General Spaces and Cartesian Spaces, (1926) Communications to the Amsterdam Academy of Sciences. English translation reprinted in Classics on Fractals, Gerald A.Edgar, editor, Addison-Wesley (1993) ISBN 0-201-58701-7
- Karl Menger, Dimensionstheorie, (1928) B.G Teubner Publishers, Lepzig.
External links
- An interactive Menger sponge (http://www.mathematik.com/Menger/Menger2.html)
- A Level 7 Menger sponge in .blend format (http://members.home.nl/dotblend/benchmark.htm)de:Menger-Schwamm
