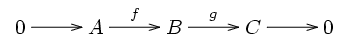Flat module
|
|
In abstract algebra, a flat module over a ring R is an R-module M such that taking the tensor product over R with M preserves exact sequences.
Vector spaces over a field are flat modules. Free modules, or more generally projective modules, are also flat, over any R. Over a Noetherian local ring, flatness, projectivity, and freeness are all equivalent.
In commutative algebra, and more generally in algebraic geometry, flatness has come to play a major role since Serre's paper Géometrie Algébrique et Géométrie Analytique. The geometric reasons are not superficial, though.
In the case when R is a commutative ring, one can say that flatness for an R-module M is equivalent to tensor product with M being an exact functor from the category of R-modules to itself. When R isn't commutative one needs the more careful statement, that (if M is a left R-module) the tensor product with M maps exact sequences of right R-modules to exact sequences of abelian groups.
Taking tensor products (over arbitrary rings) is always a right exact functor. Therefore, the R-module M is flat if and only if for any injective homomorphism K → L of R-modules, the induced homomorphism K⊗M → L⊗M is also injective.
In general, arbitrary direct sums and direct limits of flat modules are flat, a consequence of the fact that the tensor product commutes with direct sums and direct limits, and that both direct sums and direct limits are exact functors. Submodules and factor modules of flat modules need not be flat in general. However we have the following result: the homomorphic image of a flat module M is flat if and only if the kernel is a pure submodule of M.
Lazard proved in 1969 that a module M is flat if and only if it is a direct limit of finitely-generated free modules. As a consequence, one can deduce that every finitely-presented flat module is projective.
Flatness may also be expressed using the Tor functors, the left derived functors of the tensor product. A left R-module M is flat if and only if Tor1R(–, M) = 0 (i.e., iff Tor1R(X, M) = 0 for all right R-modules X). Similarly, a right R-module M is flat if and only if Tor1R(M,–) = 0. Using the Tor functor's long exact sequences, one can then easily prove facts about a short exact sequence
- If A and C are flat, then so is B
- If B and C are flat, then so is A
If A and B are flat, C need not be flat in general. However, it can be shown that
- If A is pure in B and B is flat, then A and C are flat.
See also
- localization of a module
- flat morphism
- von Neumann regular ring: those rings over which all modules are flat.