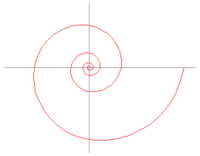
Logarithmic spiral
|
|
A logarithmic spiral, equiangular spiral or growth spiral is a special kind of spiral curve which often appears in nature. The logarithmic spiral was first described by Descartes and later extensively investigated by Jakob Bernoulli, who called it Spira mirabilis, "the marvelous spiral", and wanted one engraved on his headstone. Unfortunately, an Archimedean spiral was placed there instead.
Definition
In polar coordinates (r, θ) the curve can be written as
- <math>r = a b^\theta\ \mbox{or}\ \theta = \log_{b} (r/a)<math>, hence the name "logarithmic"
and in parametric form as
- <math>x(t) = a b^t \cos(t)<math>
- <math>y(t) = a b^t \sin(t)<math>
with positive real numbers a and b. Modifying a will rotate the spiral while b controls how tightly and in which direction it is wrapped.
The spiral is nicely parametrised in the complex plane: zt, given a z with Im(z)≠0 and |z|≠1.
In differential geometric terms the spiral can be defined as a curve c(t) having a constant angle α between the radius or path vector and the tangential vector
- <math>\arccos \frac{\langle \mathbf{c}(t), \mathbf{c}'(t) \rangle}{\|\mathbf{c}(t)\|\|\mathbf{c}'(t)\|} = \alpha <math>
If α = 0 the logarithmic spiral degenerates into a straight line. If α = ± π / 2 the logarithmic spiral degenerates into a circle.
Notes
The logarithmic spiral can be distinguished from the Archimedean spiral by the fact that the distances between the arms of a logarithmic spiral increase in geometric progression, while in an Archimedean spiral these distances are constant.
Any straight line through the origin will intersect a logarithmic spiral at the same angle α, which can be computed (in radians) as arctan(1/ln(b)). The pitch angle of the spiral is the (constant) angle the spiral makes with circles centered at the origin. It can be computed as arctan(ln(b)). A logarithmic spiral with pitch 0 degrees (b = 1) is a circle; the limiting case of a logarithmic spiral with pitch 90 degrees (b = 0 or b = ∞) is a straight line starting at the origin.
Logarithmic spirals are self-similar in that they are self-congruent under all similarity transformations (scaling them gives the same result as rotating them). Scaling by a factor <math>b^{2 \pi}<math> gives the same as the original, without rotation. They are also congruent to their own involutes, evolutes, and the pedal curves based on their centers.
Starting at a point P and moving inwards along the spiral, one has to circle the origin infinitely often before reaching it; yet, the total distance covered on this path is finite. This was first realized by Torricelli even before calculus had been invented. The total distance covered is r/cos(α), where r is the straight-line distance from P to the origin.
One can construct approximate logarithmic spirals with pitch about 17.03239 degrees using Fibonacci numbers or the golden mean as is explained in those articles. Similarly, the exponential function exactly maps all lines not parallel with the real or imaginary axis in the complex plane, to all logarithmic spirals in the complex plane with centre at 0. (Up to adding integer multiples of 2πi to the lines, the mapping of all lines to all logarithmic spirals is onto.) The pitch angle of the logarithmic spiral is the angle between the line and the imaginary plane.
Logarithmic spirals in nature
Hawks approach their prey in a logarithmic spiral: their sharpest view is at an angle to their direction of flight; this angle is the same as the spiral's pitch.
Insects approach a light source in a logarithmic spiral because they are used to having the light source at a constant angle to their flight path. Usually the sun is the only light source and flying that way will result in a practically straight line.
The arms of spiral galaxies are roughly logarithmic spirals. Our own galaxy, the Milky Way, is believed to have four major spiral arms, each of which is a logarithmic spiral with pitch of about 12 degrees.
The arms of tropical cyclones, such as hurricanes, also form logarithmic spirals.
|
Missing image |
In biology, structures approximately equal to the logarithmic spiral occur frequently, for instance in spider webs and in the shells of mollusks. The reason is the following: start with any irregularly shaped two-dimensional figure F0. Expand F0 by a certain factor to get F1, and place F1 next to F0, so that two sides touch. Now expand F1 by the same factor to get F2, and place it next to F1 as before. Repeating this will produce an approximate logarithmic spiral whose pitch is determined by the expansion factor and the angle with which the figures were placed next to each other. This is shown for polygonal figures in the accompanying graphic.