Descriptive geometry
|
|
Gaspard Monge is the father of descriptive geometry. He developed a graphical protocol which creates three-dimensional virtual space on a two dimensional plane. It builds upon a practice that evolved over centuries of displaying two images of an object, one image as seen in one direction and a second image as seen from a direction 90 degrees rotated, e.g., a "front" and a "side" view. Using Monge's protocols allows an imaginary object to be drawn such that it may be 3-D modeled, i.e., all geometric aspects of the imaginary object accounted for in true size/to-scale and shape, and then imaged as seen from any direction of space - all on a two dimensional drawing surface.
Descriptive geometry uses the image-creating technique of imaginary, parallel projectors emanating from an imaginary object and intersecting an imaginary plane of projection at right angles. The cumulative points of intersections create the desired image. See Orthographic projection, Multiviews Without Rotation.
Protocols
- Project two images of an object into mutually perpendicular, arbitrary directions. Note that by so doing each image view accommodates three dimensions of space, two dimensions displayed as full scale, mutually perpendicular axes and one as an invisible (point view) axis receding into the image space (depth). Further note that each of the two, adjacent image views shares a full-scale view of one of the three dimensions of space.
- Either of these images may serve as the beginning point for a third projected view. The third view may begin a fourth projection, and on ad infinitum. These sequential projections each represent a circuitous, 90-degree turn in space in order to view the object from a different direction.
- Each new projection utilizes a dimension in full scale that appears as point-view dimension in the previous view. To achieve the full-scale view of this dimension and accommodate it within the new view requires one to ignore the previous view and proceed to the second previous view where this dimension appears in full-scale.
- Each new view may be created by projecting into any of an infinite number of directions, perpendicular to the previous direction of projection. (Envision the many directions of the spokes of a wagon wheel each perpendicular to the direction of the axle.) The result is one of stepping circuitously about an object in 90-degree turns and viewing the object from each step. Each new view is added as an additional view to an Orthographic Projection layout display and appears in an unfolding of the glass box model.
- Aside from the Orthographic, six standard principle views (Front; Right Side; Left Side; Top; Bottom; Rear), Descriptive Geometry strives to yield three basic solution views: the true length of a line (i.e., full size, not foreshortened), the point view (end view) of a line, and the true shape of a plane (i.e., full size to scale, or not foreshortened).
Besides the intrinsic value of each of these solutions, they often serve to determine the direction of projection for the subsequent view. Per force, by the 90 degree circuitous stepping process, projecting in any direction from the point view of a line yields its true length view; projecting in a direction parallel to a true length line view yields its point view, projecting the point view of any line on a plane yields the plane's edge view; projecting in a direction perpendicular to the edge view of a plane will yield the true shape (to scale) view. These various views may be called upon to help solve engineering type problems posed by solid-geometry principles.
In addition, there is a heuristic value to studying Descriptive Geometry. Descriptive Geometry promotes visualization and spatial analytical abilities that, once mastered, are unique: it promotes one's intuitive ability to recognize the direction of viewing to best present a geometric type problem for solving. Representative examples:
The direction to best view:
- Two skew lines (pipes?) in general positions in order to determine the location of their shortest connector (common perpendicular).
- Two skew lines (pipes?) in general positions such that their shortest connector is seen in full scale.
- Two skew lines in general positions such the shortest connector parallel to a given plane is seen in full scale (say, to determine the position and the dimension of the shortest connector at a constant distance from a radiating surface).
- A plane surface such that a hole drilled perpendicular may is seen in full scale, as if looking through the hole (say, to test for clearances with other drilled holes).
- A plane equidistant from two skew lines in general positions (say, to confirm safe radiation distance?).
- The shortest distance from a point to a plane (say, to locate the most economical position for bracing).
- The line of intersection between two surfaces, including curved surfaces (say, for the most economical sizing of sections?)
- The true size of the angle between two planes.
A standard for presenting computer-modeling views analogous to orthographic, sequential projections has not yet been adopted. One candidate for such is presented in the illustrations below. The images in the illustrations were created using three-dimensional, engineering computer graphics.
Three-dimensional, computer modeling produces virtual space behind the tube, as it were, and may produce any view of a model from any direction within this virtual space. It does so without the need for adjacent orthographic views and therefore renders the circuitous, stepping protocol of Descriptive Geometry as obsolete.
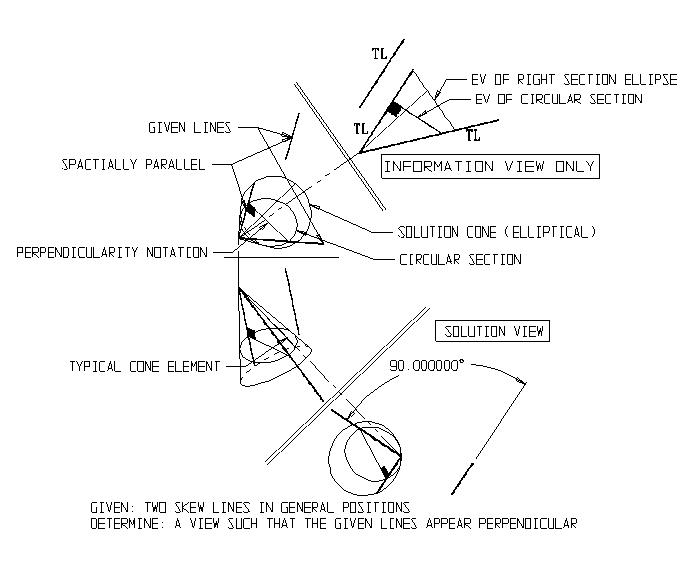
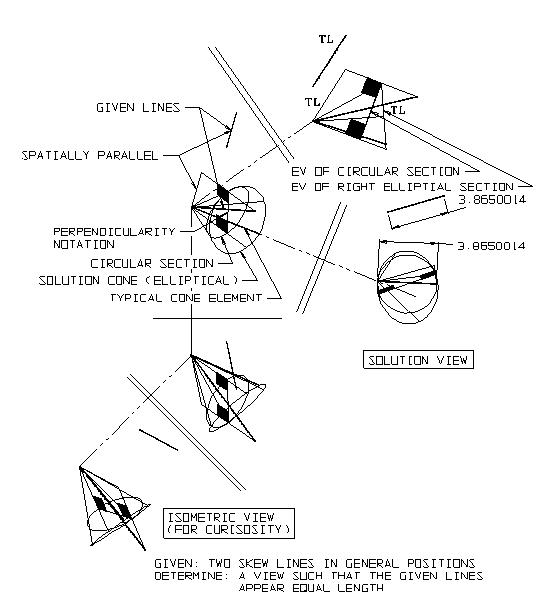
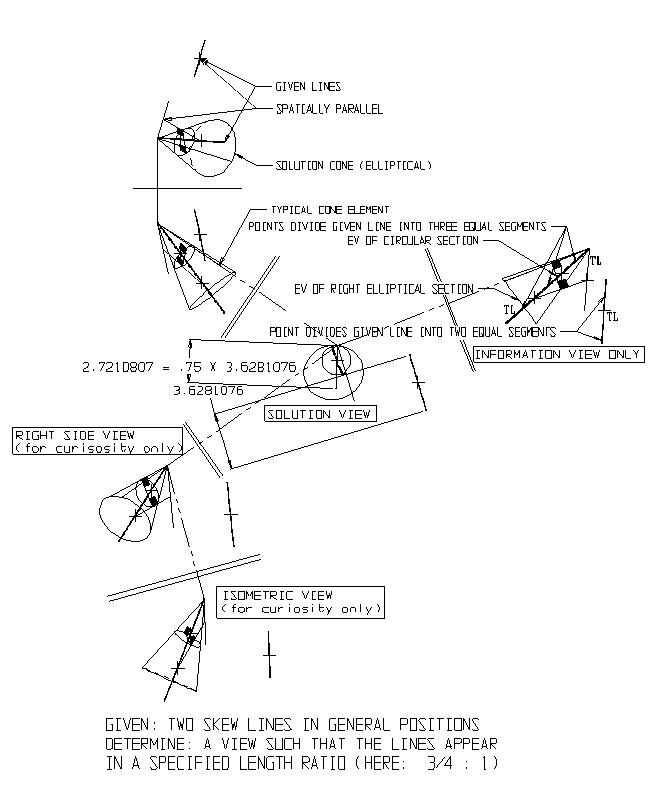
General Solutions General solutions are a class of solutions within Descriptive geometry that contain all possible solutions to a problem. The general solution is represented by a single, three-dimensional object, usually a cone, the directions of the elements of which are the desired direction of viewing (projection) for any of an infinite number of solution views.
For example: To find the general solution such that two, unequal length, skew lines in general positions (say, rockets in flight?) appear:
- Equal length
- Equal length and parallel
- Equal length and perpendicular (say, for ideal targeting of at least one)
- Equal to lengths of a specified ratio
- others.
In the examples, the general solution for each desired characteristic solution is a cone, each element of which produces one of an infinite number of solution views. When two or more characteristics of, say those listed above, are desired (and for which a solution exists) projecting in the direction of either of the two elements of intersections (one element, if cones are tangent) between the two cones produces the desired solution view. If the cones do not intersect a solution does not exist. The examples below are annotated to show the descriptive geometric principles used in the solutions. TL = True-Length; EV = Edge View.
Figs. 1-3, below, demonstrate (1) Descriptive geometry, general solutions and (2) simultaneously, a potential standard for presenting such solutions in orthographic, multiview, layout formats.
The potential standard employs two, adjacent, standard, orthographic views (here, Front and Top) with a standard "folding line" between. As there is no subsequent need to 'circuitously step 90-degrees around the object, in standard, two-step sequences in order to arrive at a solution view (it is possible to go directly to the solution view), this shorter protocol is accounted for in the layout. Where the one step protocol replaces the two-step protocol, "double folding" lines are used. In other words, when one crosses the double lines he is not making a circuitous, 90-degree turn but a non-orthodirectional turn directly to the solution view. As most engineering computer graphics packages automatically generates the six principal views of the glass box model, as well as an isometric view, these views are sometimes added out of heuristic curiosity.
Figure 1: Descriptive geometry - skew lines appearing perpendicular
Figure 2: Descriptive geometry - skew lines appear equal length
Figure 3: Descriptive geometry - skew lines appear in specified length ratio
See also: