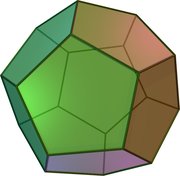
Regular polytope

A dodecahedron, one of the five Platonic solids.

In mathematics, a regular polytope is a geometric figure with a high degree of symmetry. Examples in two dimensions include the square, the regular pentagon and hexagon, and so on. In three dimensions the regular polytopes include the cube, the dodecahedron, and all Platonic solids. There exist examples in higher dimensions also. Circles and spheres, although highly symmetric, are not considered polytopes because they do not have flat faces. The strong symmetry of the regular polytopes gives them an aesthetic quality that interests both non-mathematicians and mathematicians.

Many regular polytopes, at least in two and three dimensions, exist in nature and have been known since prehistory. The earliest surviving mathematical treatment of these objects comes to us from ancient Greek mathematicians such as Euclid. Indeed, Euclid wrote a systematic study of mathematics, publishing it under the title Elements, which built up a logical theory of geometry and number theory. His work concluded with mathematical descriptions of the five Platonic solids.

The definition of the regular polytopes remained static for many centuries after Euclid. Overall however, the history of the study of regular polytopes has been one where the definition, in fits and starts, was gradually "relaxed", allowing more and more different objects to be considered among their number. The five Platonic solids were joined, towards the middle of the second millennium, by the Kepler-Poinsot polyhedra. By the end of the 19th century, mathematicians had begun to consider regular polytopes in four and higher dimensions, such as the tesseract and the 24-cell. The latter are quite hard to visualise, but still retain the aesthetically pleasing symmetry of their lower dimensional cousins. Harder still to imagine are the more modern abstract regular polytopes such as the 57-cell or the 11-cell. Mathematicians who study such objects insist, however, that the aesthetic qualities of these objects remain.

History of discovery

The history of discovery of the regular polytopes can be characterised by a gradual broadening of widespread understanding of the term. Gradually, the term "regular polytope" has been given successively wider meaning, allowing more different geometric objects to be so labeled. With each widening, new geometric figures are uncovered — these new figures usually being completely unknown to previous generations.

Prehistory

Greeks are usually credited with being the first to discover the regular polyhedra. The earliest known written records of these shapes come from Greek authors, who also gave the first known mathematical description of them.

Across the Mediterranean Sea was another civilisation, the Etruscan. There is a possibility that these people predated the Greeks in their awareness of at least some of the regular polyhedra, as evidenced by the discovery near Padua (in Northern Italy) in the late 1800s of a dodecahedron made of soapstone, and dating back more than 2,500 years (Lindemann, 1987). It may be argued, however, that the construction of this form was inspired by the pyritohedron (mentioned elsewhere in this article), as pyrite minerals are relatively abundant in that part of the world.

Even pre-dating the Etruscans however, come discoveries from Scotland of stones carved in shapes showing the symmetry of all five of the platonic solids. These stones, dating back to perhaps 4,000 years, show not only the form of each of the five platonic solids, but also the relations of duality amongst them (that is, that the centres of the faces of the cube gives the vertices of an octahedron, and so on). Examples of these stones are on display in the John Evans room (http://www.ashmol.ox.ac.uk/ash/guide/t-text/room29.html) of the Ashmolean Museum at Oxford University. It is impossible to know why these objects were made, or how the sculptor gained the inspiration for them.

There is no proof that the Etruscans or ancient Scots had any mathematical understanding of the regular solids — nor is there any proof that they did not. The root of the human discovery of the three-dimensional polytopes, particularly of the simpler ones, is probably impossible to trace. In any case, it is the Greek mathematical treatment of the platonic solids that has come down to us, and inspired our modern mathematical treatment of them.

Greeks

Some authors (Sanford, 1930) credit Pythagoras (550 BC) with being familiar with the Platonic solids, whereas others indicate that he may only have been familiar with the tetrahedron, cube, and dodecahedron, crediting the discovery of the other two to Theaetetus (an Athenian), who in any case gave a mathematical description of all five (Van der Waerden, 1954), (Euclid, book XIII). H.S.M. Coxeter (Coxeter, 1948, Section 1.9) credits Plato (400 BC) with having made models of them, and mentions that one of the earlier Pythagoreans used all five in a correspondence between the polyhedra and the nature of the universe as it was then perceived. It is from Plato's name that the term Platonic Solids is derived.

Star polyhedra

For almost 2000 years, the concept of a regular polytope remained as developed by the ancient Greek mathematicians. One might characterise the Greek definition as follows:

• A regular polygon is a (convex) planar figure with all edges equal and all corners equal
• A regular polyhedron is a solid (convex) figure with all faces being congruent regular polygons, the same number arranged around each vertex.

This definition rules out, for example, the square pyramid (since although all the faces are regular, the square base is not congruent to the triangular sides), or the shape formed by joining two tetrahedra together (since although all faces would be equilateral triangles, that is, congruent and regular, some vertices have 3 triangles and others have 4).

Finally, starting in the 15th century, the next generation of regular polytopes began to surface. The regular star polyhedra are called the Kepler-Poinsot solids, after Johannes Kepler and Louis Poinsot. They have non-convex regular polygons, typically pentagrams, either as faces or as vertex figures (circuits around each corner). Kepler's two polyhedra were constructed by others before his time, but Kepler was the first to recognise that they could be considered "regular" if one removed the restriction that regular polytopes be convex. Later, Poinsot discovered the remaining two. Cayley gave them English names which have become accepted. They are: (Kepler's) the small stellated dodecahedron and great stellated dodecahedron, and (Poinsot's) the great icosahedron and great dodecahedron.

The Kepler-Poinsot polyhedra may be constructed from the Platonic solids by a process called stellation. Most stellations are not regular. The study of stellations of the platonic solids was given a big push by H. S. M. Coxeter and others in 1938, with the now famous paper The 59 icosahedra. This work has recently been re-published (Coxeter, 1999).

The reciprocal process to stellation is called facetting (or faceting). Every stellation of one polytope is dual, or reciprocal, to some facetting of the dual polytope. The regular star polyhedra can also be obtained by facetting the Platonic solids. N. J. Bridge (1974) listed the simpler facettings of the dodecahedron, and reciprocated them to discover a stellation of the icosahedron that was missing from the famous "59". More have been discovered since, and the story is not yet ended.

Higher-dimensional polytopes

It was not until the 19th century that a Swiss mathematician, Ludwig Schläfli, examined and characterised the regular polytopes in higher dimensions. His efforts were first published in full in (Schläfli, 1901), six years posthumously, although parts of it were published in 1855 and 1858 (Schläfli, 1855), (Schläfli, 1858). Interestingly, between 1880 and 1900, Schläfli's results were rediscovered independently by at least nine other mathematicians — see (Coxeter, 1948, pp143–144) for more details.

The latter reference is probably the most comprehensive printed treatment of Schläfli's and similar results to date. Schläfli showed that there are six regular convex polytopes in 4 dimensions, and exactly three in each higher dimension. Descriptions of these may be found in the List of regular polytopes. Also of interest are the regular stellated 4-polytopes, not discovered by Schläfli. They are also described on the List of regular polytopes.

At the start of the 20th century, the definition of a regular polytope was as follows.

• A regular polygon is a polygon whose edges are all equal and whose angles are all equal.
• A regular polyhedron is a polyhedron whose faces are all congruent regular polygons, and whose vertex figures are all congruent and regular.
• And so on, a regular n-polytope is an n-dimensional polytope whose (n − 1)-dimensional faces are all regular and congruent, and whose vertex figures are all regular and congruent.

This is a "recursive" definition. It defines regularity of higher dimensional figures in terms of regular figures of a lower dimension. There is an equivalent (non-recursive) definition, which states that a polytope is regular if it has a sufficient degree of symmetry.

• An n-polytope is regular if any list consisting of:

a vertex, an edge containing it, a 2-dimensional face containing them, and so on up to n − 1 dimensions can be mapped to any other by a symmetry of the polytope.

So for example, the cube is regular because if we choose a vertex of the cube, and one of the three edges it is on, and one of the two faces containing the edge, then this triplet (vertex, edge, face) can be mapped to any other such triplet by a suitable symmetry of the cube.

Abstract regular polytopes

In the 20th century, some important developments were made. The symmetry groups of the classical regular polytopes were generalised into what are now called Coxeter groups. Coxeter groups also include the symmetry groups of regular tesselations of space or of the plane. For example, the symmetry group of an infinite chessboard would be a Coxeter group.

In the 1960s Branko Grünbaum issued a call to the geometric community to consider more abstract types of regular polytopes that he called polystromata. He developed the theory of polystromata, showing examples of new objects he called regular apeirotopes, that is, regular polytopes with infinitely many faces. A simple example of an apeirogon would be a zig-zag. It seems to satisfy the definition of a regular polygon — all the edges are the same length, and all the angles are the same. More importantly, perhaps, there are symmetries of the zig-zag that can map any pair of a vertex and attached edge to any other.

Grünbaum also discovered the 11-cell, a beautiful four-dimensional self-dual object whose facets are not icosahedra, but are "hemi-icosahedra" — that is, they are the shape one gets if one considers opposite faces of the icosahedra to be actually the same face (Grünbaum, 1977). The hemi-icosahedron has only 10 triangular faces, and 6 vertices, unlike the icosahedron, which has 20 and 12.

This concept may be easier for the reader to grasp if one considers the relationship of the cube and the hemicube. An ordinary cube has 8 corners, they could be labeled A to H, with A opposite H, B opposite G, and so on. In a hemicube, A and H would be treated as the same corner. So would B and G, and so on. The edge AB would become the same edge as GH, and the face ABEF would become the same face as CDGH. The new shape has only three faces, 6 edges and 4 corners.

A few years after Grünbaum's discovery of the 11-cell, H. S. M. Coxeter independently discovered the same shape. He had earlier discovered a similar polytope, the 57-cell (Coxeter 1982, 1984).

Unfortunately perhaps, the study of polystromata fell by the wayside, as mathematicians turned their interest to other similar abstract geometric concepts, including the concepts of buildings and geometries, abstract polytopes, Euler posets and others. The 11-cell and 57-cell remain important examples of (in particular) abstract regular polytopes.

An abstract regular polytope is defined as a set, supposed to represent the set of vertices, edges, faces and so on of a polytope, with an idea of which of these "lie on" which others. Certain restrictions are imposed on the set that are similar to properties satisfied by the classical regular polytopes (including the platonic solids). The restrictions, however, are loose enough that regular tessellations, hemicubes, and even objects as strange as the 11-cell or stranger, are all examples of regular polytopes. The theory has been developed largely by Egon Schulte and Peter McMullen (McMullen, 2002), but other researchers have also made contributions.

Constructions

Polygons

The traditional way to "construct" a regular polygon, or indeed any other figure on the plane, is by use of a ruler (more properly, a straightedge) and a compass. Constructing some regular polygons is very easy (the easiest is perhaps the equilateral triangle), some harder, or "impossible". The simplest few regular polygons that are "impossible" to construct using just a ruler and a compass are the n-sided polygons with n equal to 7, 9, 11, 13, 14, 18, 19, 21, and so forth. (See Ruler-and-compass constructions for more information.)

One thing that is commonly overlooked about ruler-and-compass constructions is that for many of the polygons, the constructions are impossible to perform using a real ruler and compass. For example, it has been shown that it is "possible" to construct a regular 65537-gon using only these tools. However, if one were to do so, making each edge 1cm long, the polygon would need to be over 200m across, and the radii of the incircle and the circumcircle would differ by less than a quarter of a micrometre — approximately the wavelength of ultraviolet light! One would need an ultraviolet camera to distinguish between this polygon and a circle — not to mention a very sharp pencil to draw it! The construction in any case would be extremely complex, and is only of theoretical interest.

Another thing that is commonly overlooked is that even "unconstructable" polygons, can be constructed, if one is satisfied with an approximation to the desired polygon, rather than with an exact representation. Indeed, with real compasses and rulers held by real hands and drawn on real paper, approximations are the best that can be achieved even for the so-called "constructable" polygons. An example that illustrates this very clearly is the following simple construction of a regular heptagon:

• Use the compass to draw a circle.
• Choose a point B on the circle.
• Without adjusting the compass, place the compass point on B, and mark two more points A and C on the circle (on opposite sides of B)
• Bisect the chord AC, to find the midpoint D
• Set the compass to distance AD
• Use the new compass setting to mark 7 points around the original circle

This construction will, to the accuracy of a typical pencil, construct a regular heptagon. If the radius of the circle is 5cm, the distance AD will be 4.3301cm. The edges of a regular heptagon should be 4.3388cm, a difference of less than 0.1mm. Very few school students, or even draftsmen, have pencils or compasses with points as sharp as that.

Polyhedra

Euclid's elements (see for example Euclid's Elements (http://www.dform.com/projects/euclid/home.html)) gave what amount to ruler-and-compass constructions for the five platonic solids. However, the merely practical question of how one might draw a straight line in space, even with a ruler, might lead one to question what exactly it means to "construct" a regular polyhedron. (One could ask the same question about the polygons, of course.)

The English word "construct" has the connotation of systematically building the thing constructed. The most common way presented to construct a regular polyhedron is via a fold-out net. To obtain a fold-out net of a polyhedron, one takes the surface of the polyhedron and cuts it along just enough edges so that the surface may be laid out flat. This gives a plan for the net of the unfolded polyhedron. Since the platonic solids have only triangles, squares and pentagons for faces, and these are all constructible with a ruler and compass, there exist ruler-and-compass methods for drawing these fold-out nets. The same applies to Kepler's polyhedra.

If this net is drawn on cardboard, or similar foldable material (for example, sheet metal), the net may be cut out, folded along the uncut edges, joined along the appropriate cut edges, and so forming the polyhedron for which the net was designed. For a given polyhedron there may be many fold-out nets. For example, there are 11 for the cube, and over 40000 for the dodecahedron. Some interesting fold-out nets of the cube, octahedron, dodecahedron and icosahedron are available here (http://www.progonos.com/furuti/MapProj/Normal/ProjPoly/projPoly.html).

Numerous children's toys, generally aimed at the teen or pre-teen age bracket, allow experimentation with regular polygons and polyhedra. For example, klikko provides sets of plastic triangles, squares, pentagons and hexagons that can be joined edge-to-edge in a large number of different ways. A child playing with such a toy could re-discover the platonic solids (or the archimedean solids), especially if given a little guidance from a knowledgeable adult.

In theory, almost any material may be used to construct regular polyhedra. Instructions for building origami models may be found here (http://hverrill.net/pages~helena/origami/pentagon/) or here (http://www1.zetosa.com.pl/~burczyk/origami/galery1-en.htm), for example. They may be carved out of wood, modeled out of wire, formed from stained glass. The imagination is the limit.

Higher dimensions

In higher dimensions, it becomes harder to say what one means by "constructing" the objects. Clearly, in a 3-dimensional universe, it is impossible to build a physical model of a 4-dimensional object. There are several approaches normally taken to overcome this matter.

The first approach is to embed the higher-dimensional objects in three-dimensional space, using methods analogous to the ways in which three-dimensional objects are drawn on the plane. For example, the fold out nets mentioned in the previous section have higher-dimensional equivalents. Some of these may be viewed at [1] (http://www.weimholt.com/andrew/polytope.shtml). One might even imagine building a model of this fold-out net, as one draws a polyhedron's fold-out net on a piece of paper. Sadly, we could never do the necessary folding of the 3-dimensional structure to obtain the 4-dimensional polytope, because of the constraints of the physical universe. Another way to "draw" the higher-dimensional shapes in 3 dimensions is via some kind of projection, for example, the analogue of either orthographic or perspective projection. Coxeter's famous book on polytopes (Coxeter, 1948) has some examples of such orthographic projections. Other examples may be found on the web (see for example [2] (http://mathworld.wolfram.com/600-Cell.html)). Note that immersing the 4-dimensional objects directly into two dimensions is quite confusing. Easier to understand are 3-d models of the projections. Such models are occasionally found in science museums or mathematics departments of universities (such as that of the Université Libre de Bruxelles).

The intersection of a four (or higher) dimensional regular polytope with a three-dimensional hyperplane will be a polytope (not necessarily regular). If the hyperplane is moved through the shape, the three-dimensional slices can be combined, animated into a kind of four dimensional object, where the fourth dimension is taken to be time. In this way, we can see (if not fully grasp) the full four-dimensional structure of the four-dimensional regular polytopes, via such cutaway cross sections. This is analogous to the way a CAT scan reassembles two-dimensional images to form a 3-dimensional representation of the organs being scanned. The ideal would be an animated hologram of some sort, however, even a simple animation such as the one shown can already give some limited insight into the structure of the polytope.

Another way a three-dimensional viewer can comprehend the structure of a four-dimensional object is through being "immersed" in the object, perhaps via some form of virtual reality technology. To understand how this might work, imagine what one would see if space were filled with cubes. The viewer would be inside one of the cubes, and would be able to see cubes in front of, behind, above, below, to the left and right of himself. If one could travel in these directions, one could explore the array of cubes, and gain an understanding of its geometrical structure. An infinite array of cubes is not a polytope in the traditional sense. In fact, it is a tessellation of 3-dimensional (Euclidean) space. However, a 4-dimensional polytope can be considered a tessellation of a 3-dimensional non-Euclidean space, namely, a tessellation of the surface of a four-dimensional sphere. Locally, this space seems like the one we are familiar with, and therefore, a virtual-reality system could, in principle, be programmed to allow exploration of these "tesselations", that is, of the 4-dimensional regular polytopes. The mathematics department at UIUC has a number of pictures of what one would see if embedded in a tessellation of hyperbolic space with dodecahedra. Such a tessellation forms an example of an infinite abstract regular polytope. An example may be seen at this page (http://www.geom.uiuc.edu/graphics/pix/Special_Topics/Hyperbolic_Geometry/HSpace.html).

Normally, for abstract regular polytopes, a mathematician considers that the object is "constructed" if the structure of its symmetry group is known. This is because of an important theorem in the study of abstract regular polytopes, providing a technique that allows the abstract regular polytope to be constructed from its symmetry group in a standard and straightforward manner.

Polytopes in nature

Polygons

The Giant's Causeway, in Ireland

Numerous regular polygons may be seen in nature. In the world of minerals, crystals often have faces which are triangular, square or hexagonal. Quasicrystals can even have regular pentagons as faces. Another fascinating example of regular polygons occurring as a result of geological processes may be seen at the Giant's Causeway in Ireland, or at the Devil's Postpile in California, where the cooling of lava has formed areas of tightly packed hexagonal columns of basalt.

Starfruit, a popular fruit in Southeast Asia

The most famous hexagons in nature are found in the animal kingdom. The wax honeycomb made by bees is an array of hexagons used to store honey and pollen, and as a secure place for the larvae to grow. There also exist animals who themselves take the approximate form of regular polygons (or at least have the same symmetry) for example starfish and sometimes other echinoderms (such as sea urchins) display the symmetry of a pentagon or sometimes other polygons (such as the heptagon). In fact, echinoderms do not display exact radial symmetry. However, Jellyfish and Comb jellies do, usually four-fold (like the square) or eight-fold.

Radial symmetry (and other symmetry) is also widely observed in the plant kingdom, particularly amongst flowers, and (to a lesser extent) seeds and fruit, the most common form of such symmetry being pentagonal. A particularly striking example is the Starfruit, a slightly tangy fruit popular in Southeast Asia, whose cross-section is shaped like a pentagonal star.

Moving off earth into space, early mathematicians doing calculations using Newton's law of gravitation discovered that if two bodies (such as the sun and the earth) are orbiting one another, there exist certain points in space where a smaller body (such as an asteroid or a space station)will remain in a stable orbit, following (for example) the earth but never catching up or falling behind. These points are called Lagrangian points. The sun-earth system has five Lagrangian points. The two most stable are exactly 60 degrees ahead and behind the earth in its orbit. That is, joining the centre of the sun and the earth and one of these stable Lagrangian points forms an equilateral triangle. Astronomers have already found asteroids at these points. It is still debated whether it is practical to keep a space station at the Lagrangian point — although it would never need course corrections, it would have to frequently dodge the asteroids that are already present there! (Already there are satellites and space observatories at the less stable Lagrangian points, which do not form the point of an equilateral triangle with the earth and the sun.)

Polyhedra

The credit for the first constructions of the platonic solids does not go to the human race — each of them occurs naturally in one form or another, although not all of these occurrences are visible to the naked eye. The tetrahedron, cube, and octahedron all occur as crystals. These by no means exhaust the numbers of possible forms of crystals (Smith, 1982, p212), of which there are 48. Neither the regular icosahedron nor the regular dodecahedron are amongst them, although one of the forms, called the pyritohedron (named for the group of minerals of which it is typical) is has twelve pentagonal faces, arranged in the same pattern as the faces of the regular dodecahedron. The faces of the pyritohedron are, however, not regular, so the pyritohedron is also not regular.

Circogonia icosahedra, a species of Radiolaria.

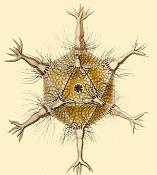
In the early 20th century, Ernst Haeckel described (Haeckel, 1904) a number of species of Radiolaria, some of whose skeletons are shaped like various regular polyhedra. Examples include Circoporus octahedrus, Circogonia icosahedra, Lithocubus geometricus and Circorrhegma dodecahedra. The shapes of these creatures should be obvious from their names.

A more recent discovery is of a series of new types of carbon molecule, known as the fullerenes (see (Curl, 1991) for an easy to read exposition of this discovery). Although C₆₀, the most easily produced fullerene, looks more or less spherical, some of the larger varieties (such as C₂₄₀, C₄₈₀ and C₉₆₀) are hypothesised to take on the form of slightly rounded icosahedra, a few nanometres across.

As an aside: In ancient times the Pythagoreans believed that there was a harmony between the regular polyhedra and the orbits of the planets. In the 17th century, Johannes Kepler studied data on planetary motion compiled by Tycho Brahe and for a decade tried to establish the pythagorean ideal by finding a match between the sizes of the polyhedra and the sizes of the planets' orbits. His search failed in its original objective, but out of this research came Kepler's discoveries of the Kepler solids as regular polytopes, the realisation that the orbits of planets are not circles, and of Kepler's laws of planetary motion for which he is now famous. In Kepler's time only five planets (excluding the earth) were known, nicely matching the number of platonic solids. Kepler's work, and the discovery since that time of Uranus, Neptune and Pluto, have thrown the Pythagorean idea well and truly into the dustbins of scientific history.

References

• (Bridge, 1974) Bridge, N. J.; Facetting the Dodecahedron Acta Crystallographica A30 pp548–552.
• (Coxeter, 1948) Coxeter, H. S. M.; Regular Polytopes, (Methuen and Co., 1948).
• (Coxeter, 1982) Coxeter, H. S. M.; Ten Toroids and Fifty-Seven hemi-Dodecahedra Geometrica Dedicata 13 pp87–99.
• (Coxeter, 1984) Coxeter, H. S. M.; A Symmetrical Arrangement of Eleven hemi-Icosahedra Annals of Discrete Mathematics 20 pp103–114.
• (Coxeter, 1999) Coxeter, H. S. M.; Du Val, P.; Flather, H. T.; Petrie, J. F.; The Fifty-Nine Icosahedra (Tarquin Publications, Stradbroke, England, 1999)
• (Curl, 1991) Curl, R. F.; Smalley, R. E.; Fullerenes, Scientific American 265 4 (1991) pp32–41.
• (Euclid) Euclid, Elements, English Translation by Heath, T. L.; (Cambridge University Press, 1956).
• (Grünbaum, 1977) Grünbaum, B.; Regularity of Graphs, Complexes and Designs, in Problèmes Combinatoires et Théorie des Graphes, Colloquium Internationale CNRS, Orsay, 260 pp191–197.
• (Haeckel, 1904) Haeckel, E.; Kunstformen der Natur (1904). Available as Haeckel, E.; Art forms in nature (Prestel USA, 1998), ISBN 3791319906, or online at http://caliban.mpiz-koeln.mpg.de/~stueber/haeckel/kunstformen/natur.html
• (Lindemann, 1987) Lindemann F.; Sitzunger Bayerische Akademie Der Wissenschaften 26 (1987) pp625–768.
• (McMullen, 2002) McMullen, P.; Schulte, S.; Abstract Regular Polytopes; (Cambridge University Press, 2002)
• (Sanford, 1930) Sanford, V.; A Short History Of Mathematics, (The Riverside Press, 1930).
• (Schläfli, 1855), Schläfli, L.; Reduction D'Une Integrale Multiple Qui Comprend L'Arc Du Cercle Et L'Aire Du Triangle Sphérique Comme Cas Particulières, Journal De Mathematiques 20 (1855) pp359–394.
• (Schläfli, 1858), Schläfli, L.; On The Multiple Integral ∫ⁿdxdy...dz, Whose Limits Are <math>p_1<math>=a₁x+b₁y+ ... +h₁z>0, <math>p_2 > 0, ... , p_n > 0<math> and <math>x^2+y^2+...+z^2<1<math> Quarterly Journal Of Pure And Applied Mathematics 2 (1858) pp269–301, 3 (1860) pp54–68, 97–108.
• (Schläfli, 1901), Schläfli, L.; Theorie Der Vielfachen Kontinuität, Denkschriften Der Schweizerischen Naturforschenden Gesellschaft 38 (1901) pp1–237.
• (Smith, 1982) Smith, J. V.; Geometrical And Structural Crystallography, (John Wiley and Sons, 1982).
• (Van der Waerden, 1954) Van der Waerden, B. L.; Science Awakening, (P Noordhoff Ltd, 1954), English Translation by Arnold Dresden.

See also

• H. S. M. Coxeter
• List of regular polytopes
• Platonic solid
• Johnson solid

External links

• Stella: Polyhedron Navigator (http://www.software3d.com/Stella.html) Tool for exploring polyhedra and printing nets
• Ernst Haeckel's Kunstformen der Natur online (German) (http://caliban.mpiz-koeln.mpg.de/~stueber/haeckel/kunstformen/natur.html)
• Interesting fold-out nets of the cube, octahedron, dodecahedron and icosahedron (http://www.progonos.com/furuti/MapProj/Normal/ProjPoly/projPoly.html)