|
|
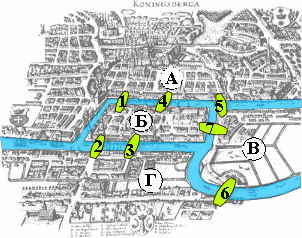
The Seven Bridges of Königsberg is a problem inspired by an actual place and situation. The city of Königsberg, Prussia (now Kaliningrad, Russia) is set on the river Pregel, and included two large islands which were connected to each other and the mainland by seven bridges. The question is whether it is possible to walk with a route that crosses each bridge exactly once, and return to the starting point. In 1736, Leonhard Euler proved that it was not possible.
Circa 1750, the prosperous and educated townspeople allegedly walked about on Sundays trying to solve the problem, but this might be an urban legend.
| Contents |
Euler's solution
In proving the result, Euler formulated the problem in terms of graph theory, by abstracting the case of Königsberg -- first, by eliminating all features except the landmasses and the bridges connecting them; second, by replacing each landmass with a dot, called a vertex or node, and each bridge with a line, called an edge or link.
 →
Missing image
→
Missing image
7_bridges.png
→
Missing image
Konigsburg_graph.png
Note that graph theory is a branch of topology. The shape of a graph may be distorted in any way, so long as the links between nodes are unchanged. It does not matter whether the links are straight or curved, or whether one node is to the left of another.
Euler showed that a circuit of the desired form is possible if and only if there are no nodes (dots in the picture of the graph) that have an odd number of edges touching them. Such a walk is called an Eulerian circuit or an Euler tour. Since the graph corresponding to Königsberg has four such nodes, the path is impossible.
If the starting point does not need to coincide with the end point there can be either zero or two nodes that have an odd number of edges touching them. Such a walk is called an Eulerian trail or Euler walk. So this too was impossible for the seven bridges of Königsberg.
Significance in the history of mathematics
In the history of mathematics, it is one of the first problems in graph theory to be formally discussed, and also, as graph theory can be seen as a part of topology, one of the first problems in topology. (The field of combinatorics also has a claim on graph theory, but combinatorial problems had been considered much earlier.)
The difference between the actual layout and the graph schematic is a good example of the idea that topology is not concerned with the rigid shape of objects.
Variations
The classic statement of the problem, given above, uses unidentified nodes -- that is, they are all alike except for the way in which they are connected. There is a variation in which the nodes are identified -- each node is given a unique name and/or color.
7_bridgesID.png
The northern bank of the river is occupied by the Schloß, or castle, of the Blue Prince; the southern by the Red Prince. The east bank is home to the Bishop's Kirche, or church; and on the small island in the center is a Gasthaus, or inn.
It is understood that the problems are taken in chronological order, and begin with a statement of the original problem:
It being customary among the townsmen, after some hours in the Gasthaus, to attempt to walk the bridges, and many have returned for more refreshment claiming success. However, none have been able to repeat the feat by the light of day.
The Blue Prince's 8th bridge
The Blue Prince, having analyzed the town's bridge system by means of graph theory, concludes the bridges cannot be walked. He contrives a stealthy plan to build an 8th bridge so that he can begin in the evening at his Schloß, walk the bridges, and end at the Gasthaus to brag of his victory. Of course, the Red Prince is unable to duplicate the feat.
- Where does the Blue Prince build the 8th bridge?
The Red Prince's 9th bridge
The Red Prince, infuriated by his brother's Gordian solution to the problem, builds a 9th bridge, enabling him to begin at his Schloß, walk the bridges, and end at the Gasthaus to rub dirt in his brother's face. His brother can then no longer walk the bridges himself.
- Where does the Red Prince build the 9th bridge?
The Bishop's 10th bridge
The Bishop has watched this furious bridge-building with dismay. It upsets the town's Weltanschauung and, worse, contributes to excessive drunkenness. He builds a 10th that allows all the inhabitants to walk the bridges and return to their own beds.
- Where does the Bishop build the 10th bridge?
Solutions
Solutions to these variant problems are given here.
See also
es:Problema de los puentes de Königsberg fr:Sept ponts de Königsberg he:הגשרים של קניגסברג lt:Septyni Karaliaučiaus tiltai hu:Königsbergi hidak nl:Zeven bruggen van Koningsbergen ja:一筆書き no:Broene i Königsberg pt:Sete pontes de Königsberg ru:Семь мостов Кёнигсберга sv:Königsbergs broproblem zh:柯尼斯堡七桥问题