Michaelis-Menten kinetics

Michaelis-Menten kinetics describe the rate of enzyme mediated reactions for many enzymes. It is named for Leonor Michaelis and Maud Menten. These kinetics are valid only when the concentration of substrate is higher than the concentration of enzyme, and in the particular case of steady-state, where the concentration of the complex enzyme-substrate is constant.

Contents

1 Determination of constants 2 Reaction rate/velocity 'V' 3 Michaelis constant 'KM' 4 Equation 5 History 6 Sources

Determination of constants

To determine the maximum rate of an enzyme mediated reaction, the substrate concentration ([S]) is increased until a constant rate of product formation is achieved. This is the maximum velocity (V_max) of the enzyme. In this state, enzyme active sites are saturated with substrate. Note that at the maximum velocity, the factors that effect the rate of enzyme mediated reactions (ie. pH, temperature, etc) are at optimal values.

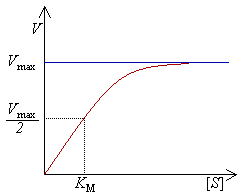
Diagram of reaction speed and Michaelis-Menten constant.

Reaction rate/velocity 'V'

The speed V means the number of reactions per second that are catalyzed by an enzyme. With increasing substrate concentration [S], the enzyme is asymptotically approaching its maximum speed V_max, but never actually reaching it. Because of that, no [S] for V_max can be given. Instead, the characteristic value for the enzyme is defined by the substrate concentration at its half-maximum speed (V_max/2). This K_M value is also called Michaelis-Menten constant.

Michaelis constant 'K_M'

Since the substrate concentration at V_max cannot be measured exactly, enzymes must be characterized by the substrate concentration at which the rate of reaction is half its maximum. This substrate concentration is called the Michaelis-Menten constant (K_M) a.k.a. Michaelis constant. This represents (for enzyme reactions exhibiting simple Michaelis-Menten kinetics) the dissociation constant (affinity for substrate) of the enzyme-substrate (ES) complex. Low values indicate that the ES complex is held together very tightly and rarely dissociates without the substrate first reacting to form product.

Equation

The derivation of "Michaelis-Menten" was actually described by Briggs and Haldane. It is obtained as follows:

The enzymatic reaction is supposed to be irreversible, and the product does not rebind the enzyme.

<math>

E + S
 \begin{matrix}
   k_1 \\
   \longrightarrow \\
   \longleftarrow  \\
   k_{-1}
 \end{matrix}
ES
 \begin{matrix}
   k_2 \\
   \longrightarrow\\
   \ 
 \end{matrix}
E + P

<math>

Because we follow the steady state approximation:

<math>\frac{d[ES]}{dt} = k_1[E][S] - k_{-1}[ES] - k_2[ES] = 0<math>

<math>[ES] = \frac{k_1[E][S]}{k_{-1} + k_2}<math>

Let's define:

<math>K_m = \frac{k_{-1} + k_2}{k_1}<math>

Therefore:

<math>[ES] = \frac{[E][S]}{K_m}<math>

And:

<math>[E] = \frac{[ES]K_m}{[S]}<math> (1)

The rate (or velocity) of the reaction is:

<math>\frac{d[P]}{dt} = k_2[ES]<math> (2)

The total concentration of enzyme is:

<math>[E_0] = [E] + [ES]<math>

Hence:

<math>[ES] = [E_0] - [E]<math> (3)

Substituting (1) in (3):

<math>[ES] = \frac{([E_0] - [ES]) [S]}{K_m}<math>

<math>[ES](1 + \frac{Km}{[S]}) = [Eo]<math>

<math>[ES] = [E_0]\frac{1}{1+\frac{K_m}{[S]}}<math> (4)

Substituting (4) in (2) and multiplying numerator and denominator by <math>[S]<math>:

<math>\frac{d[P]}{dt} = k_2[E_0]\frac{[S]}{K_m + [S]} = V_{max}\frac{[S]}{K_m + [S]} <math>

• E₀ is the total or starting amount of enzyme. It is not practical to measure the amount of the enzyme substrate complex during the reaction, so the reaction must be written in terms of the total (starting) amount of enzyme, a known quantity.
• d[P]/dt a.k.a. V₀ a.k.a. reaction velocity a.k.a. reaction rate is the rate of production of the product. Note that the term reaction velocity is misleading and reaction rate is preferred.
• k₂[E₀] a.k.a. V_max is the maximum velocity or maximum rate. k₂ is often called k_cat.

Notice that if [S] is large compared to K_m, [S]/(K_m + [S]) approaches 1. Therefore, the rate of product formation is equal to k₂[E₀] in this case.

When [S] equals K_m, [S]/(K_m + [S]) equals 0.5. In this case, the rate of product formation is half of the maximum rate (1/2 V_max). By plotting V₀ against [S], one can easily determine V_max and K_m. Note that this requires a series of experiments at constant E₀ and different substrate concentration [S].

History

The relationship between substrate concentration and enzyme concentration was proposed in 1913 by Leonor Michaelis and Maud Menten, following earlier work by Archibald Vivian Hill.

Leonor Michaelis, Maud Menten (1913). Die Kinetik der Invertinwirkung, Biochem. Z. 49:333-369.

The current derivation has been proposed by Briggs and Haldane.

G. E. Briggs and J. B. S. Haldane (1925) A note on the kinetics of enzyme action, Biochem. J., 19, 339-339.

Sources

• Catalysis chapter from the Biochemistry textbook released under the GFDL (http://textbook.wikipedia.org/wiki/Biochemistry_-_Catalysis)fr:Équation de Michaelis Menten