Cartesian coordinate system
|
|
Cartesian means relating to the French mathematician and philosopher Descartes, who, among other things, worked to merge algebra and Euclidean geometry. This work was influential to the development of analytic geometry, calculus, and cartography.
The idea of this system was developed in 1637 in two writings by Descartes. In Discourse on Method, in part two, he introduces the new idea of specifying the position of a point or object on a surface, using two intersecting axes as measuring guides. In La Géométrie, he further explores the above-mentioned concepts.
| Contents |
Two-dimensional coordinate system
The modern Cartesian coordinate system in two dimensions (also called a rectangular coordinate system) is commonly defined by two axes, at right angles to each other, forming a plane (an xy-plane). The horizontal axis is labeled x, and the vertical axis is labeled y. In a three dimensional coordinate system, another axis, normally labeled z, is added, providing a sense of a third dimension of space measurement. The axes are commonly defined as mutually orthogonal to each other (each at a right angle to the other). (Early systems allowed "oblique" axes, that is, axes that did not meet at right angles.) All the points in a Cartesian coordinate system taken together form a so-called Cartesian plane.
The point of intersection, where the axes meet, is called the origin normally labeled O. With the origin labeled O, we can name the x axis Ox and the y axis Oy. The x and y axes define a plane that can be referred to as the xy plane. Given each axis, choose a unit length, and mark off each unit along the axis, forming a grid. To specify a particular point on a two dimensional coordinate system, you indicate the x unit first (abscissa), followed by the y unit (ordinate) in the form (x,y), an ordered pair. In three dimensions, a third z unit (applicate) is added, (x,y,z).
The choices of letters come from the original convention, which is to use the latter part of the alphabet to indicate unknown values. The first part of the alphabet was used to designate known values.
An example of a point P on the system is indicated in the picture below using the coordinate (5,2).
Missing image
Cartesiancoordinates2D.JPG
Image:cartesiancoordinates2D.JPG
The arrows on the axes indicate that they extend forever in the same direction (i.e. infinitely). The intersection of the two x-y axes creates four quadrants indicated by the Roman numerals I, II, III, and IV. Conventionally, the quadrants are labeled counter-clockwise starting from the northeast quadrant. In Quadrant I the values are (x,y), and II:(-x,y), III:(-x,-y) and IV:(x,-y). (see table below.)
| Quadrant | x values | y values |
|---|---|---|
| I | > 0 | > 0 |
| II | < 0 | > 0 |
| III | < 0 | < 0 |
| IV | > 0 | < 0 |
Three-dimensional coordinate system
Sometime in the early 19th century the third dimension of measurement was added, using the z axis.
Missing image
Cartesiancoordinates3D.JPG
Image:cartesiancoordinates3D.JPG
The coordinates in a three dimensional system are of the form (x,y,z). An example of two points plotted in this system are in the picture above, points P(5, 0, 2) and Q(-5, -5, 10). Notice that the axes are depicted in a world-coordinates orientation with the z-axis pointing up.
The x, y, and z coordinates of a point (say P) can also be taken as the distances from the yz-plane, xz-plane, and xy-plane respectively. The figure below shows the distances of point P from the planes.
Missing image
Coord_planes.JPG
Image:Coord_planes.JPG
The xy-, yz-, and xz-planes divide the three-dimensional space into eight subdivisions known as octants. While conventions have been established for the labeling of the four quadrants of the x'-y plane, only the first octant of three dimensional space is labeled. It contains all of the points whose x, y, and z coordinates are positive. That is, no point in the first octant has a negative coordinate. The three dimensional coordinate system is provides the physical dimensions of space — height, width, and length, and this is often referred to as "the three dimensions". It is important to note that a dimension is simply a measure of something, and that, for each class of features to be measured, another dimension can be added. Attachment to visualizing the dimensions precludes understanding the many different dimensions that can be measured (time, mass, color, cost, etc.). It is the powerful insight of Descartes that allows us to manipulate multi-dimensional object algebraically, avoiding compass and protractor for analyzing in more than three dimensions.
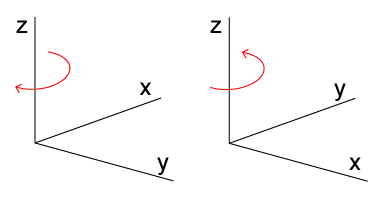
Orientation and "handedness"
The three-dimensional Cartesian coordinate system presents a problem. Once the x- and y-axes are specified, they determine the line along which the z-axis should lie, but there are two possible directions on this line. The two possible coordinate systems which result are called 'right-handed' and 'left-handed'.
The origin of these names is a trick called the right-hand rule (and the corresponding left-hand rule). If the forefinger of the right hand is pointed forward, the middle finger bent inward at a right angle to it, and the thumb placed a right angle to both, the three fingers indicate the relative directions of the x-, y-, and z-axes respectively in a right-handed system. Conversely, if the same is done with the left hand, a left-handed system results.
The right-handed system is universally accepted in the physical sciences, but the left-handed is also still in use.
If a point plotted with some coordinates in a right-handed system is replotted with the same coordinates in a left-handed system, the new point is the mirror image of the old point about the xy-plane.
Right_hand_cartesian.JPG
More ambiguity occurs when a three-dimensional coordinate system must be drawn on a two-dimensional page. Sometimes the z-axis is drawn diagonally, so that it seems to point out of the page. Sometimes it is drawn vertically, as in the above image (this is called a world coordinates orientation).
Further notes
In analytic geometry the Cartesian coordinate system is the foundation for the algebraic manipulation of geometrical shapes. Many other coordinate systems have been developed since Descartes. One common set of systems use polar coordinates; astronomers often use spherical coordinates, a type of polar coordinate system. In different branches of mathematics coordinate systems can be transformed, translated, rotated, and re-defined altogether to simplify calculation and for specialized ends.
It may be interesting to note that some have indicated that the master artists of the Renaissance used a grid, in the form of a wire mesh, as a tool for breaking up the component parts of their subjects they painted--a trade secret. That this may have influenced Descartes is merely speculative. (See perspective, projective geometry.)
References
Descartes, René. Oscamp, Paul J. (trans). Discourse on Method, Optics, Geometry, and Meteorology. 2001.
See also
External link
- Graph Paper (http://www.printfreegraphpaper.com/)bg:Декартова координатна система
cs:Kartézská soustava souřadnic de:Kartesisches Koordinatensystem eo:Kartezia Koordinato fr:Système de coordonnées cartésiennes ja:直交座標系 nl:Cartesisch coördinatenstelsel pl:Kartezjański układ współrzędnych pt:Sistema de coordenadas cartesiano ru:Прямоугольная система координат sl:Kartezični koordinatni sistem uk:Декартова система координат he:מערכת צירים קרטזית